Advertisements
Advertisements
Question
If the zeroes of the quadratic polynomial x2 + (a + 1) x + b are 2 and –3, then ______.
Options
a = –7, b = –1
a = 5, b = –1
a = 2, b = –6
a = 0, b = –6
Solution 1
If the zeroes of the quadratic polynomial x2 + (a + 1) x + b are 2 and –3, then a = 0, b = –6.
Explanation:
Given, x2 + (a + 1) x + b = 0
Sum of the zeroes = α + β = `-((a + 1))/1` = – a – 1
and product of the zeroes = αβ = `b/1` = b
Here, α = 2, β = –3
∴ 2 – 3 = –a – 1
`\implies` a = 0
and (2) (–3) = b
`\implies` b = –6
∴ a = 0 and b = –6
Solution 2
If the zeroes of the quadratic polynomial x2 + (a + 1) x + b are 2 and –3, then a = 0, b = – 6.
Explanation:
According to the question,
x2 + (a + 1)x + b
Given that, the zeroes of the polynomial = 2 and –3,
When x = 2
22 + (a + 1)(2) + b = 0
4 + 2a + 2 + b = 0
6 + 2a + b = 0
2a + b = –6 ........(1)
When x = –3,
(–3)2 + (a + 1)(–3) + b = 0
9 – 3a – 3 + b = 0
6 – 3a + b = 0
–3a + b = –6 ......(2)
Subtracting equation (2) from (1)
2a + b – (–3a + b) = –6 – (–6)
2a + b + 3a – b = –6 + 6
5a = 0
a = 0
Substituting the value of ‘a’ in equation (1), we get,
2a + b = –6
2(0) + b = –6
b = –6
RELATED QUESTIONS
The graphs of y = p(x) are given in following figure, for some polynomials p(x). Find the number of zeroes of p(x).
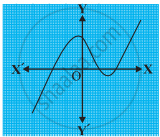
The graphs of y = p(x) are given in following figure, for some polynomials p(x). Find the number of zeroes of p(x).

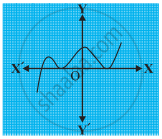
The graphs of y = p(x) are given in following figure, for some polynomials p(x). Find the number of zeroes of p(x).
Obtain all other zeroes of `(x^4 + 4x^3 – 2x^2 – 20x – 15)` if two of its zeroes are `sqrt5 and –sqrt5.`
Find all the zeroes of polynomial `(2x^4 – 11x^3 + 7x^2 + 13x – 7)`, it being given that two of its zeroes are `(3 + sqrt2) and (3 – sqrt2)`.
If 3 is a zero of the polynomial `2x^2 + x + k`, find the value of k.
If one zero of the quadratic polynomial `kx^2 + 3x + k is 2`, then find the value of k.
Find the value of k such that the polynomial x2-(k +6)x+ 2(2k - 1) has some of its zeros equal to half of their product.
If the graph of a polynomial intersects the x-axis at only one point, it cannot be a quadratic polynomial.
The zeroes of the quadratic polynomial 16x2 – 9 are ______.
