Advertisements
Advertisements
Question
In ΔABC, D and E are the mid-point on AB and AC such that DE || BC.
If AD = 8cm, AB = 12cm and AE = 12cm, find CE.
Solution
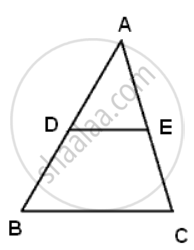
In ΔADE and ΔABC
∠D = ∠B and ∠C = ∠E ...(DE || BC)
⇒ ΔADE ∼ ΔABC
∴ `"AD"/"DB" = "AE"/"EC"`
DB
= AB - AD
= 12 - 8
= 4
⇒ `(8)/(4) = (12)/"EC"`
⇒ 8 x EC = 12 x 4
⇒ EC = `(12 xx 4)/(8)`
⇒ EC = 6cm.
APPEARS IN
RELATED QUESTIONS
In figure, ∠BAC = 90º and segment AD ⊥ BC. Prove that AD2 = BD × DC.
In the following figure, DE || OQ and DF || OR, show that EF || QR.

Show that ΔABC, where A(–2, 0), B(2, 0), C(0, 2) and ΔPQR where P(–4, 0), Q(4, 0), R(0, 2) are similar triangles
In the given figure ABC is a triangle with ∠EDB = ∠ACB. Prove that Δ ABC ~ Δ EBD. If BE = 6 cm, EC = 4 cm, BD = 5 cm. And area of Δ BED = 9 cm2. Calculate the
(1) length of AB
(2) area of Δ ABC

State, true or false:
Two isosceles-right triangles are similar.
The areas of two similar triangles ABC and PQR are in the ratio 9:16. If BC = 4.5cm, find the length of QR.
In ΔPQR, PQ = 10 cm, QR = 12cm, PR = 8 cm, find the biggest and the smallest angle of the triangle.
Two figures are similar. If the ratio of their perimeters is 8:16. What will be the ratio of the corresponding sides?
Observe the figure and complete the following activity

In fig, ∠B = 75°, ∠D = 75°
∠B ≅ [ ______ ] ...[each of 75°]
∠C ≅ ∠C ...[ ______ ]
ΔABC ~ Δ [ ______ ] ...[ ______ similarity test]
In the figure PQ || BC. If `"PQ"/"BC" = 2/5` then `"AP"/"PB"` is ______.

