Advertisements
Advertisements
Question
In ΔABC, D and E are the mid-point on AB and AC such that DE || BC.
If AD = 4x - 3, AE = 8x - 7, BD = 3x - 1 and CE = 5x - 3,Find x.
Solution
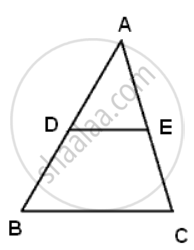
In ΔADE and ΔABC
∠D = ∠B and ∠C = ∠E ...(DE || BC)
⇒ ΔADE ∼ ΔABC
∴ `"AD"/"DB" = "AE"/"EC"`
⇒ `(4x - 3)/(3x - 1) = (8x - 7)/(5x - 3)`
⇒ (4x - 3) x (5x - 3) = (8x - 7) x (3x - 1)
⇒ 20x2 - 15x - 12x + 9 = 24x2 - 21x - 8x + 7
⇒ 20x2 - 27x + 9 = 24x2 29x + 7
⇒ 4x2 - 2x - 2 = 0
⇒ `x(x - 1) + (1)/(2)(x - 1)` = 0
⇒ `(x + 1/2)` = 0; x - 1 = 0
⇒ x = `-(1)/(2); x = 1`
∴ x = 1.
APPEARS IN
RELATED QUESTIONS
State, true or false:
Two isosceles-right triangles are similar.
The two similar triangles are equal in area. Prove that the triangles are congruent.
In the given figure, if ∠ADE = ∠B, show that ΔADE ~ ΔABC. If AD = 3.8cm, AE = 3.6cm, BE = 2.1cm and BC = 4.2cm, find DE.
The perimeter of two similar triangles ABC and PQR are 32cm and 24cm respectively. If PQ = 12cm, find AB.
In the following figure, seg BE ⊥ seg AB and seg BA ⊥ seg AD. If BE = 6 and \[\text{AD} = 9 \text{ find} \frac{A\left( \Delta ABE \right)}{A\left( \Delta BAD \right)} \cdot\]

In the given figure, ∠ABC = 75°, ∠EDC = 75° state which two triangles are similar and by which test? Also write the similarity of these two triangles by a proper one to one correspondence.

On a map drawn to a scale of 1 : 25000, a rectangular plot of land, ABCD is measured as AB= 12 cm and BC = 16cm. calculate the diagonal distance of the plot in km and the plot area in km2 .
In the given figure, PQ || AB; CQ = 4.8 cm QB = 3.6 cm and AB = 6.3 cm. Find : If AP = x, then the value of AC in terms of x.

In ΔABC, DE is drawn parallel to BC cutting AB in the ratio 2 : 3. Calculate:
(i) `("area"(Δ"ADE"))/("area"(Δ"ABC")`
(i) `("area"("trapeziumEDBC"))/("area"(Δ"ABC"))`
Write the test of similarity for triangles given in figure.
