Advertisements
Advertisements
Question
In a ΔABC, if L and M are points on AB and AC respectively such that LM || BC. Prove
that:
(1) ar (ΔLCM ) = ar (ΔLBM )
(2) ar (ΔLBC) = ar (ΔMBC)
(3) ar (ΔABM) ar (ΔACL)
(4) ar (ΔLOB) ar (ΔMOC)
Solution
(1) Clearly Triangles LMB and LMC are on the same base LM and between the same
parallels LM and BC.
∴ ar (ΔLMB) = ar (ΔLMC) ......(1)
(2) We observe that triangles LBC and MBC area on the same base BC and between the
same parallels LM and BC
∴ arc ΔLBC = ar (MBC) ..........(2)
(3) We have
ar (ΔLMB) = ar (ΔLMC) [from (1)]
⇒ ar ( ΔALM) + ar (ΔLMB) = ar (ΔALM) + ar (LMC)
⇒ ar (ΔABM) = ar (ΔACL)
(4) We have
ar(ΔCBC) = ar (ΔMBC) ∴ [from (1)]
⇒ ar (ΔLBC) = ar (ΔBOC) = a (ΔMBC) - ar (BOC)
⇒ ar (ΔLOB) = ar (ΔMOC)
APPEARS IN
RELATED QUESTIONS
Show that the diagonals of a parallelogram divide it into four triangles of equal area.
In the given figure, diagonals AC and BD of quadrilateral ABCD intersect at O such that OB = OD. If AB = CD, then show that:
(i) ar (DOC) = ar (AOB)
(ii) ar (DCB) = ar (ACB)
(iii) DA || CB or ABCD is a parallelogram.
[Hint: From D and B, draw perpendiculars to AC.]

In the given figure, ABCDE is a pentagon. A line through B parallel to AC meets DC produced at F. Show that
(i) ar (ACB) = ar (ACF)
(ii) ar (AEDF) = ar (ABCDE)

Diagonals AC and BD of a quadrilateral ABCD intersect each other at P. Show that ar (APB) × ar (CPD) = ar (APD) × ar (BPC).
[Hint : From A and C, draw perpendiculars to BD.]
ABC and BDE are two equilateral triangles such that D is the mid-point of BC. Then ar (BDE) = `1/4` ar (ABC).
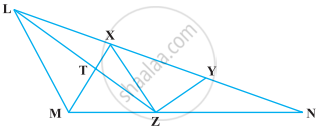
X and Y are points on the side LN of the triangle LMN such that LX = XY = YN. Through X, a line is drawn parallel to LM to meet MN at Z (See figure). Prove that ar (LZY) = ar (MZYX)
The area of the parallelogram ABCD is 90 cm2 (see figure). Find ar (ΔABD)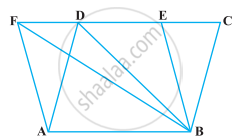
The area of the parallelogram ABCD is 90 cm2 (see figure). Find ar (ΔBEF)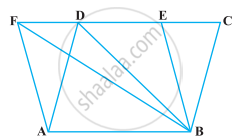
In ∆ABC, D is the mid-point of AB and P is any point on BC. If CQ || PD meets AB in Q (Figure), then prove that ar (BPQ) = `1/2` ar (∆ABC).
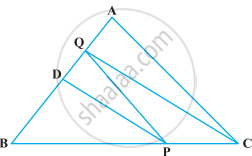
O is any point on the diagonal PR of a parallelogram PQRS (Figure). Prove that ar (PSO) = ar (PQO).