Advertisements
Advertisements
Question
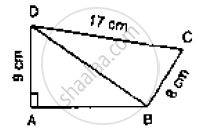
In the below figure, compute the area of quadrilateral ABCD.
Solution
Given that
DC = 17cm
AD = 9cm and BC = 8cm
In ΔBCD we have
CD2 = BD 2 + BC2
⇒ (17)2 = BD2 + (8)2
⇒ BD2 = 289 - 64
⇒ BD = 15
In ΔABD, we have
AB2 + AD2 = BD2
⇒ (15)2 = AB2 + (9)2
⇒ AB2 = 225 - 81 = 144
⇒ AB = 12
ar ( quad , ABCD) =ar ( ΔABD) + ar ( Δ BCD)
⇒ ar ( quad , ABCD) = `1/2` ( 12 × 9 ) + `1/2` (8 × 17 ) = 54 + 68
= 112cm2
⇒ ar (quad, , ABCD =`1/2`(12 × 9 ) +`1/2 ( 8 × 15 )`
= 54 + 60cm2
= 114cm2
APPEARS IN
RELATED QUESTIONS
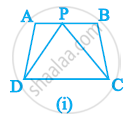
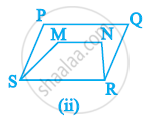
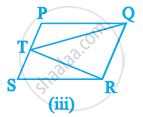
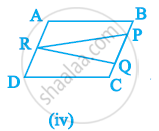
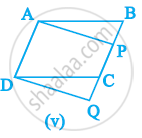
Which of the following figures lie on the same base and between the same parallels. In such a case, write the common base and the two parallels.

In the below figure, PQRS is a square and T and U are respectively, the mid-points of PS
and QR. Find the area of ΔOTS if PQ = 8 cm.

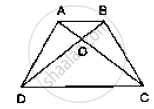
In the below fig. ABCD is a trapezium in which AB || DC. Prove that ar (ΔAOD) =
ar(ΔBOC).
In Fig. below, CD || AE and CY || BA.
(i) Name a triangle equal in area of ΔCBX
(ii) Prove that ar (Δ ZDE) = ar (ΔCZA)
(iii) Prove that ar (BCZY) = ar (Δ EDZ)

In the below fig. ABCD is a trapezium in which AB || DC and DC = 40 cm and AB = 60
cm. If X and Y are respectively, the mid-points of AD and BC, prove that:
(i) XY = 50 cm
(ii) DCYX is a trapezium
(iii) ar (trap. DCYX) =`9/11`ar (trap. (XYBA))
In the below figure, ABCD is parallelogram. O is any point on AC. PQ || AB and LM ||
AD. Prove that ar (||gm DLOP) = ar (||gm BMOQ)
If bisectors of ∠A and ∠B of a quadrilateral ABCD meet at O, then ∠AOB is
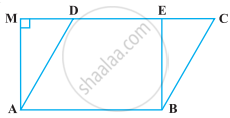
In the following figure, if parallelogram ABCD and rectangle ABEM are of equal area, then ______.
ABCD is a quadrilateral whose diagonal AC divides it into two parts, equal in area, then ABCD ______.
