Advertisements
Advertisements
Question
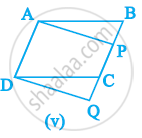
Which of the following figures lie on the same base and between the same parallels. In such a case, write the common base and the two parallels.
Solution
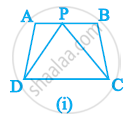
Yes. It can be observed that trapezium ABCD and triangle PCD have a common base CD and these are lying between the same parallel lines AB and CD.
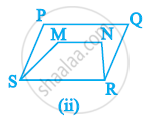
No. It can be observed that parallelogram PQRS and trapezium MNRS have a common base RS. However, their vertices, (i.e., opposite to the common base) P, Q of parallelogram and M, N of trapezium, are not lying on the same line.
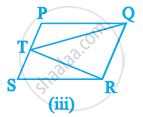
Yes. It can be observed that parallelogram PQRS and triangle TQR have a common base QR and they are lying between the same parallel lines PS and QR.
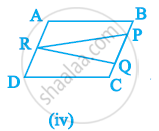
No. It can be observed that parallelogram ABCD and triangle PQR are lying between same parallel lines AD and BC. However, these do not have any common base.
Yes. It can be observed that parallelogram ABCD and parallelogram APQD have a common base AD and these are lying between the same parallel lines AD and BQ.
No. It can be observed that parallelogram PBCS and PQRS are lying on the same base PS. However, these do not lie between the same parallel lines.
APPEARS IN
RELATED QUESTIONS
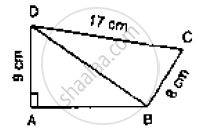
In the below figure, compute the area of quadrilateral ABCD.
In the below figure, PQRS is a square and T and U are respectively, the mid-points of PS
and QR. Find the area of ΔOTS if PQ = 8 cm.
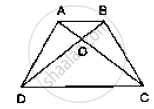
In the below fig. ABCD is a trapezium in which AB || DC. Prove that ar (ΔAOD) =
ar(ΔBOC).
In Fig. below, CD || AE and CY || BA.
(i) Name a triangle equal in area of ΔCBX
(ii) Prove that ar (Δ ZDE) = ar (ΔCZA)
(iii) Prove that ar (BCZY) = ar (Δ EDZ)
In the below fig. ABCD is a trapezium in which AB || DC and DC = 40 cm and AB = 60
cm. If X and Y are respectively, the mid-points of AD and BC, prove that:
(i) XY = 50 cm
(ii) DCYX is a trapezium
(iii) ar (trap. DCYX) =`9/11`ar (trap. (XYBA))
In the below figure, ABCD is parallelogram. O is any point on AC. PQ || AB and LM ||
AD. Prove that ar (||gm DLOP) = ar (||gm BMOQ)
If bisectors of ∠A and ∠B of a quadrilateral ABCD meet at O, then ∠AOB is
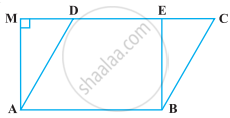
In the following figure, if parallelogram ABCD and rectangle ABEM are of equal area, then ______.
ABCD is a quadrilateral whose diagonal AC divides it into two parts, equal in area, then ABCD ______.
