Question

In fig., AB and DC are two chords of a circle with centre O. these chords when produced meet at P. if PB = Bern, BA = 7cm and PO = 14.5cm, find the radius of the circle. 

Answer 1

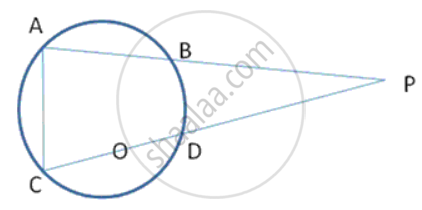
Let OD = OC = r (say) 

PO = 14.5 , CP = r + 14.5 

PD = 14.5 - r 

In Δ BPD and Δ APC 

∠ BPD = ∠ APC {Common) 

∠ ABD + ∠ DBP = 180° ---(1) ( Linear pair ) 

Also, ∠ ABD + ∠ ACD = 180° ---(2} {Opposite angles of a cyclic quadrilateral) 

From (1) and {2} 

∠ DBP = ∠ ACD 

∴ Δ BPD ~ Δ CPA    {AA corollary) 

`8/("r" + 14.5) = (4.5 - "r")/15`

120° = 14.5² - r² 

^r2 = 210.25- 120

r² = 90.25

r = 9.50 

Radius of the circle is 9.5cm .