Advertisements
Advertisements
Question
Prove that the tangent at any point of a circle is perpendicular to the radius through the point of contact.
Solution 1
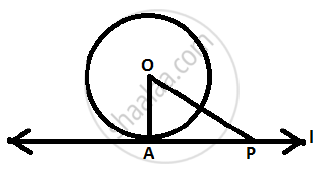
Given: Line is tangent to the (O, r) at point A.
To prove: `bar(OA) _|_ l`
Proof: Let P ∈ l , P ≠ A.
If P is in the interior of (O,r), then the line will be a secant of the circle and not a tangent.
But l is a tangent of the circle, so P is not in the interior of the circle.
Also P≠A
P is the point in the exterior of the circle.
OP > OA ( `bar(OA)` is the radius of the circle )
Therefore each point P ∈ l except A satisfies the inequality OP > OA.
Therefore OA is the shortest distance of line l from O.
`bar(OA) _|_ l`
Solution 2

Given: A circle C (0, r) and a tangent l at point A
To prove: OA ⊥ l
Construction:
Take a point B, other than A, on the tangent l. Join OB. Suppose OB meets the circle in C.
Proof: We know that among all line segments joining the point O to a point on l, the perpendicular is shortest to l.
OA = OC (Radius of the same circle)
Now,
OB = OC + BC
⇒ OB > OC
⇒ OB > OA
⇒ OA < OB
B is an arbitrary point on the tangent l. Thus, OA is shorter than any other line segment joining O to any point on l.
Here,
OA ⊥ l
Hence, the tangent at any point of a circle is perpendicular to the radius through the point of contact.
APPEARS IN
RELATED QUESTIONS
How many tangents can a circle have?
In the given figure, PA and PB are tangents to the circle from an external point P. CD is another tangent touching the circle at Q. If PA = 12 cm, QC = QD = 3 cm, then find PC + PD
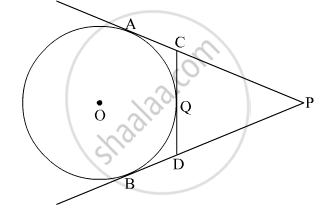
In following fig., PT is tangent to the circle at T and CD is a diameter of the same circle. If PC= 3cm and PT= 6cm, find the radius of the circle.

Calculate the length of direct common tangent to two circles of radii 3cm and Bern with their centres 13cm apart.
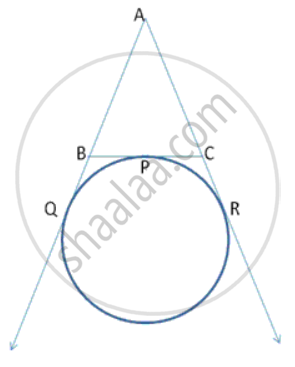
In following fig., a circle is touching the side BC of Δ ABC at P and AB and AC produced at Q and R respectively. Prove that AQ is half the perimeter of Δ ABC.
In the given figure, diameter AB and chord CD of a circle meet at P. PT is a tangent to the circle at T. CD = 7.8 cm, PD = 5 cm, PB = 4 cm. Find the length of tangent PT.

In the figure given below, PT is a tangent to the circle. Find PT if AT = 16 cm and AB = 12 cm.

In the alongside, figure, O is the centre of the circumcircle of triangle XYZ. Tangents at X and Y intersect at T. Given ∠XTY = 80° and ∠XOZ = 140°. Calculate the value of ∠ZXY.

In the following figure, PA and PB are tangents from a point P to a circle with centre O. Then the quadrilateral OAPB must be a ______

In the given figure, PT is a tangent at T to the circle with centre O. If ∠TPO = 25°, then x is equal to ______.

