Advertisements
Advertisements
Question
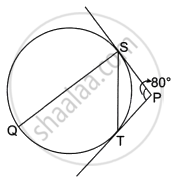
In the alongside, figure, O is the centre of the circumcircle of triangle XYZ. Tangents at X and Y intersect at T. Given ∠XTY = 80° and ∠XOZ = 140°. Calculate the value of ∠ZXY.

Solution
∠ TXY = ∠ TYX = 50° ...(since XT = YT)
∠ OXZ = ∠ OZX = 20° ...(since OX = OZ)( In ΔXOZ, 140° + ∠ OXZ + ∠OZX = 180°)
⇒ ∠ OXZ = 20°
⇒ ∠ OXY = 40° ...(Since ∠OXT = 90°)
⇒ ∠ZXY = ∠ OXZ + ∠ OXY
= 20° + 40° = 60°.
APPEARS IN
RELATED QUESTIONS
In the figure given below, PQ is a chord of a circle with centre O and PT is a tangent. If ∠QPT = 60°, find ∠PRQ.

Prove that the tangent at any point of a circle is perpendicular to the radius through the point of contact.
The given figure shows a circle with centre O and BCD is tangent to it at C. Show that : ∠ACD + ∠BAC = 90°.

In the following figure, PQ is the tangent to the circle at A, DB is a diameter and O is the centre of the circle. If ∠ ADB = 30° and ∠ CBD = 60° ; calculate : ∠CDB

In a square ABCD, its diagonal AC and BD intersect each other at point O. The bisector of angle DAO meets BD at point M and bisector of angle ABD meets AC at N and AM at L. Show that - ALOB is a cyclic quadrilateral.
Two circle with radii r1 and r2 touch each other externally. Let r be the radius of a circle which touches these two circle as well as a common tangent to the two circles, Prove that: `1/sqrtr + 1/sqrtr_1 + 1/sqrtr_2`.
In figure, M is the centre of the circle and seg KL is a tangent segment. If MK = 12, KL = `6sqrt(3)`, then find
(i) Radius of the circle.
(ii) Measures of ∠K and ∠M.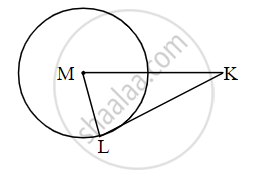
In the following figure, PA and PB are tangents from a point P to a circle with centre O. Then the quadrilateral OAPB must be a ______

If two tangents TL and TM are drawn to a circle with centre C such that ∠LTM = 70°, then find ∠MCT.

In the given diagram, PS and PT are the tangents to the circle. SQ || PT and ∠SPT = 80°. The value of ∠QST is ______.