Advertisements
Advertisements
Question
In the given figure, DE║BC such that AD = x cm, DB = (3x + 4) cm, AE = (x + 3) cm and EC = (3x + 19) cm. Find the value of x.
Solution
In ΔADE and ΔABC
∠𝐴𝐷𝐸 = ∠𝐴𝐵𝐶 (𝐶𝑜𝑟𝑟𝑒𝑠𝑝𝑜𝑛𝑑𝑖𝑛𝑔 𝑎𝑛𝑔𝑙𝑒𝑠 𝑖𝑛 𝐷𝐸 ∥ 𝐵𝐶)
∠𝐴𝐸𝐷 = ∠𝐴𝐶𝐵 (𝐶𝑜𝑟𝑟𝑒𝑠𝑝𝑜𝑛𝑑𝑖𝑛𝑔 𝑎𝑛𝑔𝑙𝑒𝑠 𝑖𝑛 𝐷𝐸 ∥ 𝐵𝐶
By AA similarity criterion, ΔADE ~ ΔABC
If two triangles are similar, then the ratio of their corresponding sides are proportional
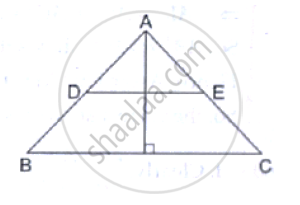
∴ `(AD)/(AB)=(AE)/(AC)`
⇒`( AD)/(AD+DB)=(AE)/(AE+EC)`
⇒` x/(x+3x+4)=(x+3)/(x+3+3x+19)`
⇒ `x/(4x+4)=(x+3)/(x+3+3x+19)`
⇒ `x/(2x+2)=(x+3)/(2x+11)`
⇒ `2x^2+11x=2x^2+2x+6x+6`
⇒ 3x=6
⇒ x=2
Hence, the value of x is 2.
APPEARS IN
RELATED QUESTIONS
State which pair of triangles in the following figure are similar. Write the similarity criterion used by you for answering the question, and also write the pairs of similar triangles in the symbolic form:

In the following figure, altitudes AD and CE of ΔABC intersect each other at the point P. Show that:
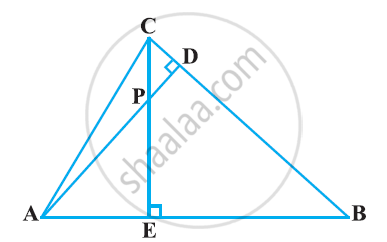
ΔAEP ∼ ΔADB
In a right angled triangle with sides a and b and hypotenuse c, the altitude drawn on the hypotenuse is x. Prove that ab = cx.
The sides of certain triangles are given below. Determine which of them right triangles are.
7cm, 24cm, 25cm
Two triangles DEF an GHK are such that ∠D = 48° and ∠H = 57° . If ΔDEF ∼GHK then find the measures of ∠F
In the given figure, value of x(in cm) is

In figure, BD and CE intersect each other at the point P. Is ΔPBC ~ ΔPDE? Why?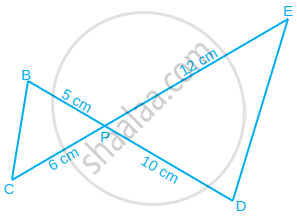
If in triangles ABC and DEF, `(AB)/(DE) = (BC)/(FD)`, then they will be similar, when ______.
In the given figure below, `(AD)/(AE) = (AC)/(BD)` and ∠1 = ∠2, Show that ΔBAE ∼ ΔCAD.

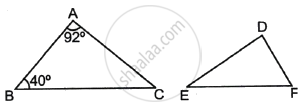
If ΔABC ∼ ΔDEF such that ∠A = 92° and ∠B = 40°, then ∠F = ?