Advertisements
Advertisements
Question
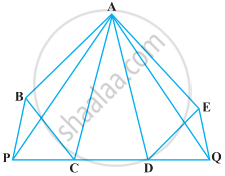
In the following figure, ABCDE is any pentagon. BP drawn parallel to AC meets DC produced at P and EQ drawn parallel to AD meets CD produced at Q. Prove that ar (ABCDE) = ar (APQ)
Solution
Given: ABCDE is a pentagon.
BP || AC and EQ|| AD.
To prove: ar (ABCDE) = ar (APQ)
Proof: We know that, triangles on the same base and between the same parallels are equal in area.
Here, ΔADQ and ΔADE lie on the same base AD and between the same parallels AD and EQ.
So, ar (ΔADQ) = ar (ΔADE) ...(i)
Similarly, ΔACP and ΔACB lie on the same base AC and between the same parallels AC and BP.
So, ar (ΔACP) = ar (ΔACB) ...(ii)
On adding equations (i) and (ii), we get
ar (ΔADQ) + ar (ΔACP) = ar (ΔADE) + ar (ΔACB)
On adding ar (ΔACD) both sides, we get
ar (ΔADQ) + ar (ΔACP) + ar (ΔACD) = ar (ΔADE) + ar (ΔACB) + ar (ΔACD)
⇒ ar (ΔAPQ) = ar (ABCDE)
Hence proved.
APPEARS IN
RELATED QUESTIONS
D, E and F are respectively the mid-points of the sides BC, CA and AB of a ΔABC. Show that
(i) BDEF is a parallelogram.
(ii) ar (DEF) = 1/4ar (ABC)
(iii) ar (BDEF) = 1/2ar (ABC)
D and E are points on sides AB and AC respectively of ΔABC such that
ar (DBC) = ar (EBC). Prove that DE || BC.
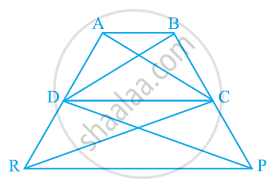
In the given figure, ar (DRC) = ar (DPC) and ar (BDP) = ar (ARC). Show that both the quadrilaterals ABCD and DCPR are trapeziums.
P and Q are respectively the mid-points of sides AB and BC of a triangle ABC and R is the mid-point of AP, show that
(i) ar(PRQ) = 1/2 ar(ARC)
(ii) ar(RQC) = 3/8 ar(ABC)
(iii) ar(PBQ) = ar(ARC)
In a ΔABC, P and Q are respectively the mid-points of AB and BC and R is the mid-point
of AP. Prove that :
(1) ar (Δ PBQ) = ar (Δ ARC)
(2) ar (Δ PRQ) =`1/2`ar (Δ ARC)
(3) ar (Δ RQC) =`3/8` ar (Δ ABC) .
ABCD is a parallelogram and X is the mid-point of AB. If ar (AXCD) = 24 cm2, then ar (ABC) = 24 cm2.
In the following figure, ABCD and EFGD are two parallelograms and G is the mid-point of CD. Then ar (DPC) = `1/2` ar (EFGD).
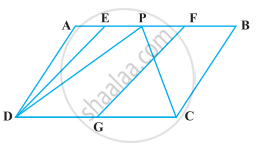
A point E is taken on the side BC of a parallelogram ABCD. AE and DC are produced to meet at F. Prove that ar (ADF) = ar (ABFC)
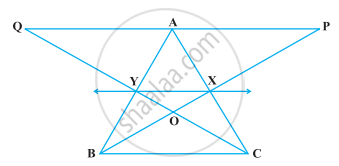
In the following figure, CD || AE and CY || BA. Prove that ar (CBX) = ar (AXY).

In the following figure, X and Y are the mid-points of AC and AB respectively, QP || BC and CYQ and BXP are straight lines. Prove that ar (ABP) = ar (ACQ).