Advertisements
Advertisements
Question
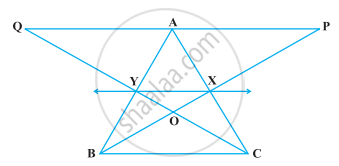
In the following figure, X and Y are the mid-points of AC and AB respectively, QP || BC and CYQ and BXP are straight lines. Prove that ar (ABP) = ar (ACQ).
Solution
Given: X and Y are the mid-points of AC and AB respectively. Also, QP || BC and CYQ, BXP are straight lines.
To prove: ar (ΔABP) = ar (ΔACQ)
Proof: Since, X and Y are the mid-points of AC and AB respectively.
So, XY || BC
We know that, triangles on the same base and between the same parallels are equal in area.
Here, ΔBYC and ΔBXC lie on same base BC and between the same parallels BC and XY.
So, ar (ΔBYC) = ar (ΔBXC)
On subtracting ar (ΔBOC) from both sides, we get
ar (ΔBYC) – ar (ΔBOC) = ar (ΔBXC) – ar (ΔBOC)
=» ar (ΔBOY) = ar (ΔCOX)
On adding ar (ΔXOY) both sides, we get
ar (ΔSOY) + ar (ΔXOY) = ar (ΔCOX) + ar (ΔXOY)
⇒ ar (ΔBYX) = ar (ΔCXY) ...(i)
Hence, we observe that quadrilaterals XYAP and YXAQ are on the same base XY and between the same parallels XY and PQ.
ar (XYAP) = ar (YXAQ) ...(ii)
On adding equations (i) and (ii), we get
ar (ΔBYX) + ar (XYAP) = ar (ΔCXY) + ar (YXAQ)
⇒ ar (ΔABP) = ar (ΔACQ)
Hence proved.
APPEARS IN
RELATED QUESTIONS
XY is a line parallel to side BC of a triangle ABC. If BE || AC and CF || AB meet XY at E and F respectively, show that
ar (ABE) = ar (ACF)
Diagonals AC and BD of a trapezium ABCD with AB || DC intersect each other at O. Prove that ar (AOD) = ar (BOC).
ABCD is a trapezium with AB || DC. A line parallel to AC intersects AB at X and BC at Y. Prove that ar (ADX) = ar (ACY).
[Hint: Join CX.]
In the given figure, AP || BQ || CR. Prove that ar (AQC) = ar (PBR).

P and Q are respectively the mid-points of sides AB and BC of a triangle ABC and R is the mid-point of AP, show that
(i) ar(PRQ) = 1/2 ar(ARC)
(ii) ar(RQC) = 3/8 ar(ABC)
(iii) ar(PBQ) = ar(ARC)
PQRS is a parallelogram whose area is 180 cm2 and A is any point on the diagonal QS. The area of ∆ASR = 90 cm2.
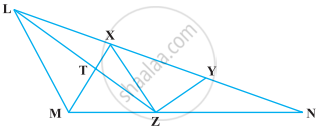
X and Y are points on the side LN of the triangle LMN such that LX = XY = YN. Through X, a line is drawn parallel to LM to meet MN at Z (See figure). Prove that ar (LZY) = ar (MZYX)
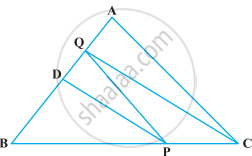
In ∆ABC, D is the mid-point of AB and P is any point on BC. If CQ || PD meets AB in Q (Figure), then prove that ar (BPQ) = `1/2` ar (∆ABC).
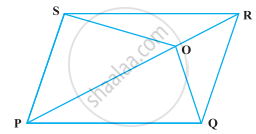
O is any point on the diagonal PR of a parallelogram PQRS (Figure). Prove that ar (PSO) = ar (PQO).
If the medians of a ∆ABC intersect at G, show that ar (AGB) = ar (AGC) = ar (BGC) = `1/3` ar (ABC)
