Advertisements
Advertisements
Question
A point E is taken on the side BC of a parallelogram ABCD. AE and DC are produced to meet at F. Prove that ar (ADF) = ar (ABFC)
Solution
Given in the question, A point E is taken on the side BC of a parallelogram ABCD. AE and DC are produced to meet at F.
Prove that ar (ADF) = ar (ABFC)
Proof: ABCD is a parallelogram and AC divides it into two triangle of equal area.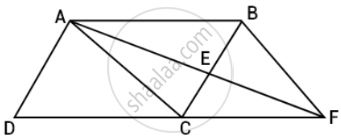
ar (ΔADC) = ar (ΔABC) ...(I)
So, DC || AB and CF || AB
As we know that triangle on the same base and between the same parallels are equal in area.
So, ar (ΔACF) = ar (ΔBCF) ...(II)
Adding equation (I) and (II), we get
ar (ΔADC) + ar (ACF) = ar (ΔABC) + ar (ΔBCF)
ar (ΔADF) = ar (ABFC)
Hence proved.
APPEARS IN
RELATED QUESTIONS
In the given figure, E is any point on median AD of a ΔABC. Show that ar (ABE) = ar (ACE)
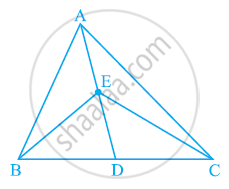
In a triangle ABC, E is the mid-point of median AD. Show that ar (BED) = 1/4ar (ABC).
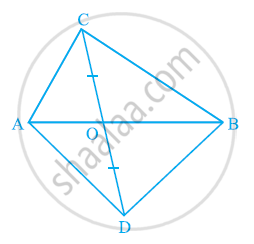
In the given figure, ABC and ABD are two triangles on the same base AB. If line-segment CD is bisected by AB at O, show that ar (ABC) = ar (ABD).
XY is a line parallel to side BC of a triangle ABC. If BE || AC and CF || AB meet XY at E and F respectively, show that
ar (ABE) = ar (ACF)
Diagonals AC and BD of a quadrilateral ABCD intersect each other at P. Show that ar (APB) × ar (CPD) = ar (APD) × ar (BPC).
[Hint : From A and C, draw perpendiculars to BD.]
ABCD is a parallelogram and X is the mid-point of AB. If ar (AXCD) = 24 cm2, then ar (ABC) = 24 cm2.
The area of the parallelogram ABCD is 90 cm2 (see figure). Find ar (ΔABD)
The medians BE and CF of a triangle ABC intersect at G. Prove that the area of ∆GBC = area of the quadrilateral AFGE.
If the medians of a ∆ABC intersect at G, show that ar (AGB) = ar (AGC) = ar (BGC) = `1/3` ar (ABC)
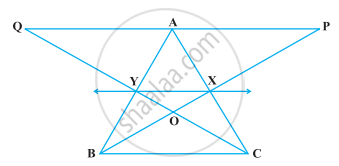
In the following figure, X and Y are the mid-points of AC and AB respectively, QP || BC and CYQ and BXP are straight lines. Prove that ar (ABP) = ar (ACQ).