Advertisements
Advertisements
Question
In the given figure, AB ∥ SQ ∥ DC and AD ∥ PR ∥ BC. If the area of quadrilateral ABCD is 24 square units, find the area of quadrilateral PQRS.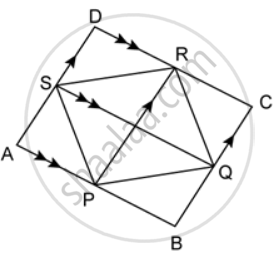
Solution
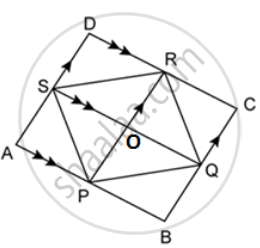
Let SQ and PR intersect at point O.
Now,
DC || SQ and RP || BCC
⇒ RC || OQ and RO || QC
⇒ QuadrilateralROQC is a parallelogram.
Similarly,
Quadrilateral ROSD, APOS and POQB are parallelograms.
ΔROQ and parallelogram ROQC are on the same base and between the same parallel lines.
∴ A(ΔROQ) = `(1)/(2)` x A(||gm ROQC) .....(i)
Similarly,
A(ΔPOQ) = `(1)/(2)` x A(||gm POQB) .....(ii)
A(ΔPOS) = `(1)/(2)` x A(||gm APOS) .....(iii)
A(ΔSOR) = `(1)/(2)` x A(||gm ROSD) .....(iv)
Adding equations (i), (ii), (iii) and (iv), we get
A(ΔROQ) + A(ΔPOQ) + A(ΔPOS) + A(ΔSOR)
= `(1)/(2)`[A(||gm ROQC) + A(||gm POQB) + A(||gm APOS) + A(||gm ROSD)]
⇒ A(||gm PQRS)
= `(1)/(2)` x A(||gm ABCD)
= `(1)/(2) xx 24`
= 12 sq. units.
APPEARS IN
RELATED QUESTIONS
Prove that if the diagonals of a parallelogram are equal then it is a rectangle.
P is a point on side KN of a parallelogram KLMN such that KP : PN is 1 : 2. Q is a point on side LM such that LQ : MQ is 2 : 1. Prove that KQMP is a parallelogram.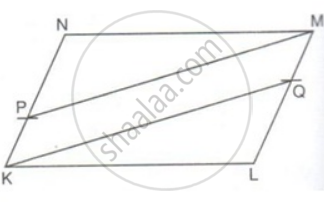
Prove that the line segment joining the mid-points of the diagonals of a trapezium is parallel to each of the parallel sides, and is equal to half the difference of these sides.
In a parallelogram PQRS, M and N are the midpoints of the opposite sides PQ and RS respectively. Prove that
PMRN is a parallelogram.
In the given figure, PQRS is a parallelogram in which PA = AB = Prove that: SA ‖ QB and SA = QB.
In the given figure, PQRS is a parallelogram in which PA = AB = Prove that: SAQB is a parallelogram.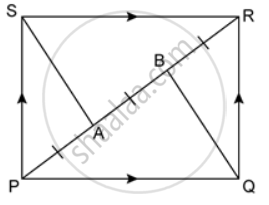
In the given figure, PQRS is a trapezium in which PQ ‖ SR and PS = QR. Prove that: ∠PSR = ∠QRS and ∠SPQ = ∠RQP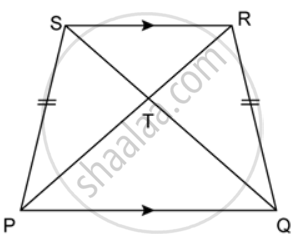
Prove that the diagonals of a kite intersect each other at right angles.
In ΔABC, the mid-points of AB, BC and AC are P, Q and R respectively. Prove that BQRP is a parallelogram and that its area is half of ΔABC.
In the figure, ABCD is a parallelogram and CP is parallel to DB. Prove that: Area of OBPC = `(3)/(4)"area of ABCD"`