Advertisements
Advertisements
Question
Let Δ be the area of a triangle. Find the area of a triangle whose each side is twice the side of the given triangle.
Solution
We are given assumed value Δ is the area of a given triangle ABC
We assume the sides of the given triangle ABC be a, b, c
The area of a triangle having sides a, b, c and s as semi-perimeter is given by,
`A = sqrt(s(s-a)(s-b)(s-c))`
`Δ = sqrt( s(s-a)(s-b)(s-c))` ...................(1)
Where,
`s =(a+b+c)/2`
`2s = a +b+c` ....................(2)
We take the sides of a new triangle as 2a, 2b, 2c that is twice the sides of previous one
Now, the area of a triangle having sides 2a, 2b, and 2c and s1 as semi-perimeter is given by,
`A_1 = sqrt(s_1(s_1-2a)(s_1-2b)(s_1-2c))`, where
`s_1 = ( 2a+2b+2c)/2`
`s_1 = (2(a+b+c))/2`
s1 = a+ b+ c
s1 =2s ..................(using (2))
Now,
`A_1 = sqrt(2s(2s-2a)(2s-2b)(2s-2c)`
`A_1= sqrt(2x xx 2 (s-a) xx 2 (s-b) xx 2(s-c))`
`A_1 = 4sqrt(4(s-a)(s-b)(s-c))`
`A_1 = 4Δ` ...................(using (1))
APPEARS IN
RELATED QUESTIONS
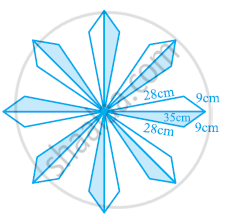
A floral design on a floor is made up of 16 tiles which are triangular, the sides of the triangle being 9 cm, 28 cm and 35 cm (see the given figure). Find the cost of polishing the tiles at the rate of 50p per cm2.
Two parallel side of a trapezium are 60 cm and 77 cm and other sides are 25 cm and 26 cm. Find the area of the trapezium.
Find the area of a rhombus whose perimeter is 80 m and one of whose diagonal is 24 m.
Find the area of a quadrilateral ABCD in which AD = 24 cm, ∠BAD = 90° and BCD forms an equilateral triangle whose each side is equal to 26 cm. (Take √3 = 1.73)
Mark the correct alternative in each of the following:
The sides of a triangle are 16 cm, 30 cm, 34 cm. Its area is
The base of an isosceles right triangle is 30 cm. Its area is
The sides of a triangle are 7 cm, 9 cm and 14 cm. Its area is
If the area of an isosceles right triangle is 8 cm2, what is the perimeter of the triangle?
The area of an equilateral triangle with side `2sqrt(3)` cm is ______.
The edges of a triangular board are 6 cm, 8 cm and 10 cm. The cost of painting it at the rate of 9 paise per cm2 is ______.
