Advertisements
Advertisements
Question
Let \[A = \left\{ x: x \in N \right\}, B = \left\{ x: x - 2n, n \in N \right\}, C = \left\{ x: x = 2n - 1, n \in N \right\}\] and D = {x : x is a prime natural number}. Find: \[A \cap D\]
Solution
\[A = \left\{ x: x \in N \right\} = {1, 2, 3, . . . }\]
\[B = \left\{ x: x - 2n, n \in N \right\} = {2, 4, 6, 8, . . . } \]
\[C = \left\{ x: x = 2n - 1, n \in N \right\} = {1, 3, 5, 7, . . . }\]
D = {x:x is a prime natural number.} = {2, 3, 5, 7,...}
\[A \cap D\]
APPEARS IN
RELATED QUESTIONS
Express the truth of each of the following statements by Venn diagram:
(a) Some hardworking students are obedient.
(b) No circles are polygons.
(c) All teachers are scholars and scholars are teachers.
In a survey it was found that 21 people liked product A, 26 liked product B and 29 liked product C. If 14 people liked products A and B, 12 people liked products C and A, 14 people liked products B and C and 8 liked all the three products. Find how many liked product C only.
Draw a Venn diagram for the truth of the following statement :
All rational number are real numbers.
Draw Venn diagram for the truth of the following statements :
Some rectangles are squares.
If A and B are two sets such that \[A \subset B\] then find:
\[A \cup B\]
If A = {1, 2, 3, 4, 5}, B = {4, 5, 6, 7, 8}, C = {7, 8, 9, 10, 11} and D = {10, 11, 12, 13, 14}, find:
\[A \cup B\]
If A = {1, 2, 3, 4, 5}, B = {4, 5, 6, 7, 8}, C = {7, 8, 9, 10, 11} and D = {10, 11, 12, 13, 14}, find:
\[B \cup C\]
If A = {1, 2, 3, 4, 5}, B = {4, 5, 6, 7, 8}, C = {7, 8, 9, 10, 11} and D = {10, 11, 12, 13, 14}, find:
\[B \cup C \cup D\]
Let \[A = \left\{ x: x \in N \right\}, B = \left\{ x: x - 2n, n \in N \right\}, C = \left\{ x: x = 2n - 1, n \in N \right\}\]and D = {x : x is a prime natural number}. Find: \[A \cap B\]
Let \[A = \left\{ x: x \in N \right\}, B = \left\{ x: x - 2n, n \in N \right\}, C = \left\{ x: x = 2n - 1, n \in N \right\}\] and D = {x : x is a prime natural number}. Find: \[A \cap C\]
Let \[A = \left\{ x: x \in N \right\}, B = \left\{ x: x - 2n, n \in N \right\}, C = \left\{ x: x = 2n - 1, n \in N \right\}\] and D = {x : x is a prime natural number}. Find: \[B \cap C\]
Let \[A = \left\{ x: x \in N \right\}, B = \left\{ x: x - 2n, n \in N \right\}, C = \left\{ x: x = 2n - 1, n \in N \right\}\] and D = {x : x is a prime natural number}. Find: \[C \cap D\]
Let A = {3, 6, 12, 15, 18, 21}, B = {4, 8, 12, 16, 20}, C = {2, 4, 6, 8, 10, 12, 14, 16} and D = {5, 10, 15, 20}. Find: \[A - B\]
Let A = {3, 6, 12, 15, 18, 21}, B = {4, 8, 12, 16, 20}, C = {2, 4, 6, 8, 10, 12, 14, 16} and D = {5, 10, 15, 20}. Find: \[A - D\]
Represent the union of two sets by Venn diagram for the following.
Y = {y | y is an odd number between 90 and 100}
Express the truth of each of the following statements using Venn diagrams:
(a) No circles are polygons
(b) Some quadratic equations have equal roots
Express the truth of each of the following statements by Venn diagram :
(a) Some hardworking students are obedient.
(b) No circles are polygons.
(c) All teachers are scholars and scholars are teachers.
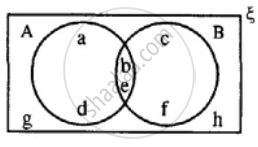
From the given diagram find :
(A ∪ B)'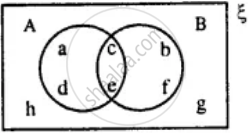
Use the given Venn-diagram to find :
A ∩ B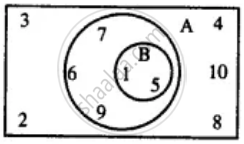
Use the given Venn-diagram to find :
A ∪ B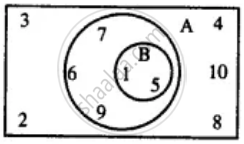
Draw a Venn-diagram to show the relationship between two overlapping sets A and B. Now shade the region representing :
A ∩ B
Draw a Venn-diagram to show the relationship between two sets A and B; such that A ⊆ B, Now shade the region representing :
A ∪ B
Draw a Venn-diagram to show the relationship between two sets A and B; such that A ⊆ B, Now shade the region representing :
B' ∩ A
Draw a Venn-diagram to show the relationship between two sets A and B; such that A ⊆ B, Now shade the region representing :
(A ∪ B)'
State the sets representing by the shaded portion of following venn-diagram :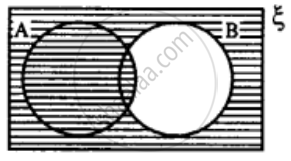
In the given diagram, shade the region which represents the set given underneath the diagrams: (B - A)'

In the given diagram, shade the region which represents the set given underneath the diagrams: (A ∩ B)'
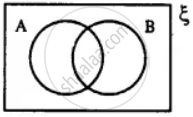
In the given diagram, shade the region which represents the set given underneath the diagrams: (P ∩ Q)'
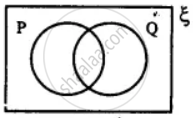
Using the given diagram, express the following sets in the terms of A and B. {a, d}
Represent the truth of the following statement by the Venn diagram.
No circles are polygons.
Draw a Venn diagram for the truth of the following statement.
Some share brokers are chartered accountants.
Draw a Venn diagram for the truth of the following statement.
No wicket keeper is bowler, in a cricket team.
Represent the following statement by the Venn diagram.
No circle is rectangle.
Draw Venn diagram for the following:
No policeman is thief
Take the set of natural numbers from 1 to 20 as universal set and show set X using Venn diagram.
X = {x | x ∈ N, and 7 < x < 15}
