Advertisements
Advertisements
Question
Δ ABC is similar to Δ PQR. If AB = 6cm, BC = 9cm, PQ = 9cm and PR = 10.5cm, find the lengths of AC and QR.
Solution
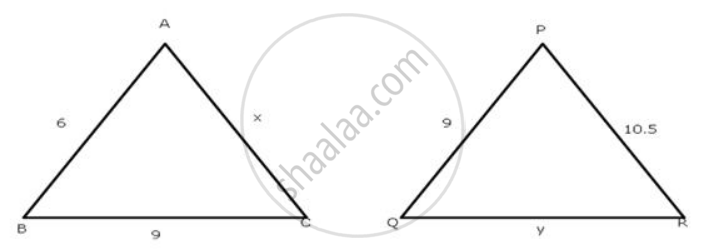
Given : Δ ABC ∼ Δ PQR
To find : AC and QR
Sol : Δ ABC ∼ Δ PQR
`therefore "AB"/"PQ" = "BC"/"QR" = "AC"/"PR"` (Similar sides of similar triangles)
`6/9 = 9/"y" = "x"/10.5`
`6/9 = 9/"y"` `6/9 = "x"/10.5`
⇒ 6y = 81 ⇒ 63 = 9x
⇒ y = `81/6` ⇒ x = 7
⇒ y = `27/2` ⇒ AC = 7 cm
⇒ QR = 13.5 cm
APPEARS IN
RELATED QUESTIONS
Show that ΔABC, where A(–2, 0), B(2, 0), C(0, 2) and ΔPQR where P(–4, 0), Q(4, 0), R(0, 2) are similar triangles
The given diagram shows two isosceles triangles which are similar. In the given diagram, PQ and BC are not parallel; PC = 4, AQ = 3, QB = 12, BC = 15 and AP = PQ.

Calculate:
- the length of AP,
- the ratio of the areas of triangle APQ and triangle ABC.
In the given figure, BC is parallel to DE. Area of triangle ABC = 25 cm2, Area of trapezium BCED = 24 cm2 and DE = 14 cm. Calculate the length of BC. Also, find the area of triangle BCD.

Two chords AB and CD of a circle intersect at a point P outside the circle.
Prove that: (i) Δ PAC ~ Δ PDB (ii) PA. PB = PC.PD

ΔABC~ΔPQR and ar(ΔABC) = 4, ar(ΔPQR) . If BC = 12cm, find QR.
In the given figure, ABCD is a trapezium with AB || DC, AB = 18 cm, DC = 32 cm and the distance between AB and AC is 14 cm. If arcs of equal radii 7 cm taking A, B, C and D as centres, have been drawn, then find the area of the shaded region ?

In ΔABC, AB = 8cm, AC = 10cm and ∠B = 90°. P and Q are the points on the sides AB and AC respectively such that PQ = 3cm ad ∠PQA = 90. Find: The area of ΔAQP.
ΔABC is enlarged, with a scale factor 5. Find: A'B', if AB = 4cm
A model of cargo tuck is made to a scale of 1:40. The length of the model is 15cm. Calculate: The length of the truck
In the given figure the value of x is

