Advertisements
Advertisements
Question
Obtain an expression for a time period of the simple pendulum.
Solution
Expression for a time period of the simple pendulum:
- Let ‘m’ be the mass of the bob and T′ be the tension in the string. The pendulum remains in equilibrium in the position OA, with the center of gravity of the bob, vertically below the point of suspension O.
- If now the pendulum is displaced through a small angle θ, called angular amplitude, and released, it begins to oscillate on either side of the mean (equilibrium) position in a single vertical plane.
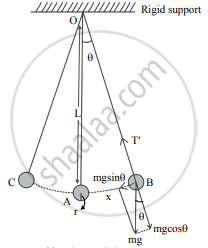
Simple pendulum - In the displaced position (extreme position), two forces are acting on the bob.
a. Force T′ due to tension in the string, directed along the string, towards the support.
b. Weight mg, in the vertically downward direction. - At the extreme positions, there should not be any net force along the string.
- The component of mg can only balance the force due to tension. Thus, weight mg is resolved into two components;
a. The component mg cosθ along the string, which is balanced by the tension T′.
b. The component mg sinθ perpendicular to the string is the restoring force acting on mass m tending to return it to the equilibrium position.
∴ Restoring force, F = –mg sinθ - As θ is very small (θ <10°),
sinθ ≈ θc
∴ F ≈ –mgθ
From the figure,
For small angle, θ = `"x"/"L"`
∴ F = -mg`"x"/"L"` ........(1)
As m, g and L are constant, F ∝ –x - Thus, for small displacement, the restoring force is directly proportional to the displacement and is oppositely directed. Hence the bob of a simple pendulum performs linear S.H.M. for small amplitudes.
- The period T of oscillation of a pendulum is given by,
T = `(2pi)/ω = (2pi)/sqrt("acceleration per unit displacement")`
Using equation (1),
F = -mg`"x"/"L"`
∴ ma = -mg`"x"/"L"` .............(∵ F = ma)
∴ a = -g`"x"/"L"`
∴ `"a"/"x" = -"g"/"L" = "g"/"L"` (in magnitude)
Substituting in the expression for T,
T = `2pisqrt("L"/"g")`
This gives the expression for the time period of a simple pendulum.
APPEARS IN
RELATED QUESTIONS
Obtain the expression for the period of a simple pendulum performing S.H.M.
Answer in brief.
State the law of simple pendulum.
A simple pendulum of length 100 cm performs S.H.M. Find the restoring force acting on its bob of mass 50 g when the displacement from the mean position is 3 cm.
A simple pendulum of length I has a bob of mass m. It executes SHM of small amplitude A. The maximum tension in the string is (g = acceleration due to gravity)
The length of a simple pendulum is increased by 44%. The percentage change in its time period is ______
Work done in stretching a wire through 1 mm is 2 J. What amount of work will be done for elongating another wire of same material, with half the length and double the radius of cross-section, by 1 mm?
A simple pendulum has a time period T1 when on the earth's surface and T2 when taken to a height R above the earth's surface, where R is the radius of the earth. The value of T2 / T1 is ______.
Two pendulums begin to swing simultaneously. The first pendulum makes nine full oscillations when the other makes seven. The ratio of the lengths of the two pendulums is ______.
Two light balls are suspended as shown in figure. When a stream of air passes through the space between them, the distance between the balls will ______.
The period of simple pendulum is measured as T in a stationary lift. If the lift moves upward with an acceleration of 5 g, the period will ____________.
If a pendulum which gives correct time beats second on ground at a certain place is moved to the top of a tower 320 m high, then the loss of time of the pendulum clock in one day, in second, is ______.
A person measures a time period of a simple pendulum inside a stationary lift and finds it to be T. If the lift starts accelerating upwards with an acceleration `("g"/3)`, the time period of the pendulum will be ______.
A pendulum performs S.H.M. with period `sqrt3` second in a stationary lift. If lift moves up with acceleration `"g"/3`, the period of pendulum is ______. (g = acceleration due to gravity)
A simple pendulum has length 'L1'. When its amplitude is 'a' cm, its energy is 'E1'. When length is 2L1, its energy is 'E2' Amplitude is same for both the lengths The relation between E1 and E2 is ______.
The length of a seconds pendulum is 1m on the earth. If the mass and diameter of the planet is double than that of the earth, then the length of the second's pendulum on the planet will be ______
Define second's pendulum.
The maximum frequency of transmitted radio waves above which the radio waves are no longer reflected back by ionosphere is ______.
(N = maximum electron density of lonosphere, g = acceleration due to gravity)
The bob of a simple pendulum performs SHM with period T in air and with period T1 in water Relation between T and T1 is ______.
(neglect friction due to water, density of the material of the bob is = `9/8xx10^3` kgm3, density of water = 1 gcc-1)
The ratio of frequencies of two oscillating pendulums is 3 : 2. Their lengths are in the ratio.
A pendulum bob is swinging in a vertical plane, such that its angular amplitude is less than 90°. At its highest point, the string is cut. Which trajectory is possible for bob afterwards?
Two weights w1 and w2 are suspended from the ends of light string over a smooth fixed pulley. If the pulley is pulled up with acceleration g, the tension in the string will be ______.
The equation of motion in differential form for a seconds pendulum is ______.
A simple pendulum of length 196 cm performs linear SHM of amplitude 8 cm. Find the restoring force on its bob of mass 50 g at an extreme position.
The velocity of bob of a second’s pendulum when it is 6 cm from its mean position and amplitude of 10 cm, is ______.
