Advertisements
Advertisements
Question
“Politics is the art of the possible”. Similarly, “Science is the art of the soluble”. Explain this beautiful aphorism on the nature and practice of science.
Solution 1
Science is a systematised study of observations. A scientist patiently analyses these observations and comes out with certain laws. As an illustration, Tycho Brahe worked for twenty long years to make observations on planetary motions. It is from this huge reservoir of observations that Kepler formulated his three famous laws of planetary motion. Thus, science is the art of the soluble just as politics is the art of the possible.
Solution 2
It is well known that to win over votes, politicians would make anything and everything possible even when they are least sure of the same. and in Science the various natural phenomena can be explained in terms of some basic laws. So as 'Politics is the art of possible' similarly 'Science is the art of the soluble'.
APPEARS IN
RELATED QUESTIONS
India has had a long and unbroken tradition of great scholarship — in mathematics, astronomy, linguistics, logic and ethics. Yet, in parallel with this, several superstitious and obscurantistic attitudes and practices flourished in our society and unfortunately continue even today — among many educated people too. How will you use your knowledge of science to develop strategies to counter these attitudes ?
Suppose a quantity x can be dimensionally represented in terms of M, L and T, that is, `[ x ] = M^a L^b T^c`. The quantity mass
Choose the correct statements(s):
(a) All quantities may be represented dimensionally in terms of the base quantities.
(b) A base quantity cannot be represented dimensionally in terms of the rest of the base quantities.
(c) The dimensions of a base quantity in other base quantities is always zero.
(d) The dimension of a derived quantity is never zero in any base quantity.
Find the dimensions of Planck's constant h from the equation E = hv where E is the energy and v is the frequency.
Can you add two vectors representing physical quantities having different dimensions? Can you multiply two vectors representing physical quantities having different dimensions?
Let \[\vec{a} = 4 \vec{i} + 3 \vec{j} \text { and } \vec{b} = 3 \vec{i} + 4 \vec{j}\]. Find the magnitudes of (a) \[\vec{a}\] , (b) \[\vec{b}\] ,(c) \[\vec{a} + \vec{b} \text { and }\] (d) \[\vec{a} - \vec{b}\].
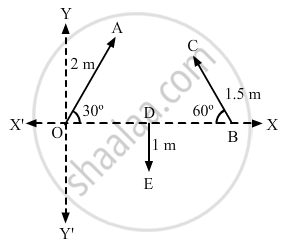
Refer to figure (2 − E1). Find (a) the magnitude, (b) x and y component and (c) the angle with the X-axis of the resultant of \[\overrightarrow{OA}, \overrightarrow{BC} \text { and } \overrightarrow{DE}\].
If \[\vec{A} , \vec{B} , \vec{C}\] are mutually perpendicular, show that \[\vec{C} \times \left( \vec{A} \times \vec{B} \right) = 0\] Is the converse true?
A curve is represented by y = sin x. If x is changed from \[\frac{\pi}{3}\text{ to }\frac{\pi}{3} + \frac{\pi}{100}\] , find approximately the change in y.
If π = 3.14, then the value of π2 is ______
