Advertisements
Advertisements
Question
PQRS is a rectangle inscribed in a quadrant of a circle of radius 13 cm. A is any point on PQ. If PS = 5 cm, then ar (PAS) = 30 cm2.
Options
True
False
Solution
This statement is True.
Explanation:
Given, PS = 5 cm
Radius of circle = SQ = 13 cm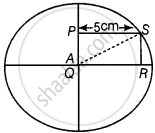
In right-angled ΔSPQ,
SQ2 = PQ2 + PS2 ...[By Pythagoras theorem]
(13)2 = PQ2 + (5)2
⇒ PQ2 = 169 – 25 = 144
⇒ PQ = 12 cm ...[Taking positive square root, because length is always positive]
Now, area of ΔAPS = `1/2` × Base × Height
= `1/2 xx PS xx PQ`
= `1/2 xx 5 xx 12`
= 30 cm2
So, given statement is true, if A coincides Q.
APPEARS IN
RELATED QUESTIONS
If E, F, G and H are respectively the mid-points of the sides of a parallelogram ABCD show that ar (EFGH) = 1/2ar (ABCD)
Parallelogram ABCD and rectangle ABEF are on the same base AB and have equal areas. Show that the perimeter of the parallelogram is greater than that of the rectangle.
In the following figure, ABCD is parallelogram and BC is produced to a point Q such that AD = CQ. If AQ intersect DC at P, show that
ar (BPC) = ar (DPQ).
[Hint: Join AC.]

In the given below fig. ABCD, ABFE and CDEF are parallelograms. Prove that ar (ΔADE)
= ar (ΔBCF)
Two parallelograms are on equal bases and between the same parallels. The ratio of their areas is ______.
ABCD is a parallelogram in which BC is produced to E such that CE = BC (Figure). AE intersects CD at F. If ar (DFB) = 3 cm2, find the area of the parallelogram ABCD.
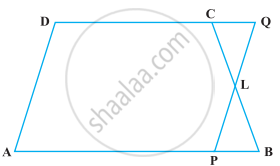
In trapezium ABCD, AB || DC and L is the mid-point of BC. Through L, a line PQ || AD has been drawn which meets AB in P and DC produced in Q (Figure). Prove that ar (ABCD) = ar (APQD)
If the mid-points of the sides of a quadrilateral are joined in order, prove that the area of the parallelogram so formed will be half of the area of the given quadrilateral (Figure).
[Hint: Join BD and draw perpendicular from A on BD.]

The diagonals of a parallelogram ABCD intersect at a point O. Through O, a line is drawn to intersect AD at P and BC at Q. Show that PQ divides the parallelogram into two parts of equal area.
In the following figure, ABCD and AEFD are two parallelograms. Prove that ar (PEA) = ar (QFD). [Hint: Join PD].

