Advertisements
Advertisements
Question
In the following figure, ABCD is parallelogram and BC is produced to a point Q such that AD = CQ. If AQ intersect DC at P, show that
ar (BPC) = ar (DPQ).
[Hint: Join AC.]

Solution
It is given that ABCD is a parallelogram.
AD || BC and AB || DC(Opposite sides of a parallelogram are parallel to each other)
Join point A to point C.
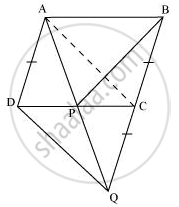
Consider ΔAPC and ΔBPC
ΔAPC and ΔBPC are lying on the same base PC and between the same parallels PC and AB. Therefore,
Area (ΔAPC) = Area (ΔBPC) ... (1)
In quadrilateral ACDQ, it is given that
AD = CQ
Since ABCD is a parallelogram,
AD || BC (Opposite sides of a parallelogram are parallel)
CQ is a line segment which is obtained when line segment BC is produced.
∴ AD || CQ
We have,
AC = DQ and AC || DQ
Hence, ACQD is a parallelogram.
Consider ΔDCQ and ΔACQ
These are on the same base CQ and between the same parallels CQ and AD. Therefore,
Area (ΔDCQ) = Area (ΔACQ)
⇒ Area (ΔDCQ) − Area (ΔPQC) = Area (ΔACQ) − Area (ΔPQC)
⇒ Area (ΔDPQ) = Area (ΔAPC) ... (2)
From equations (1) and (2), we obtain
Area (ΔBPC) = Area (ΔDPQ)
APPEARS IN
RELATED QUESTIONS
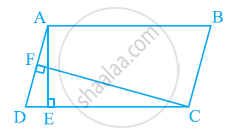
In the given figure, ABCD is parallelogram, AE ⊥ DC and CF ⊥ AD. If AB = 16 cm, AE = 8 cm and CF = 10 cm, find AD.
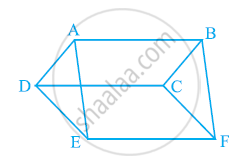
In the following figure, ABCD, DCFE and ABFE are parallelograms. Show that ar (ADE) = ar (BCF).
In which of the following figures, you find two polygons on the same base and between the same parallels?
Two parallelograms are on equal bases and between the same parallels. The ratio of their areas is ______.
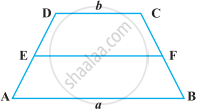
ABCD is a trapezium with parallel sides AB = a cm and DC = b cm (Figure). E and F are the mid-points of the non-parallel sides. The ratio of ar (ABFE) and ar (EFCD) is ______.
In the following figure, PSDA is a parallelogram. Points Q and R are taken on PS such that PQ = QR = RS and PA || QB || RC. Prove that ar (PQE) = ar (CFD).

ABCD is a parallelogram in which BC is produced to E such that CE = BC (Figure). AE intersects CD at F. If ar (DFB) = 3 cm2, find the area of the parallelogram ABCD.
If the mid-points of the sides of a quadrilateral are joined in order, prove that the area of the parallelogram so formed will be half of the area of the given quadrilateral (Figure).
[Hint: Join BD and draw perpendicular from A on BD.]

The diagonals of a parallelogram ABCD intersect at a point O. Through O, a line is drawn to intersect AD at P and BC at Q. Show that PQ divides the parallelogram into two parts of equal area.
In the following figure, ABCD and AEFD are two parallelograms. Prove that ar (PEA) = ar (QFD). [Hint: Join PD].

