Advertisements
Advertisements
Question
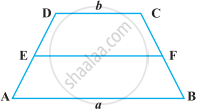
ABCD is a trapezium with parallel sides AB = a cm and DC = b cm (Figure). E and F are the mid-points of the non-parallel sides. The ratio of ar (ABFE) and ar (EFCD) is ______.
Options
a : b
(3a + b) : (a + 3b)
(a + 3b) : (3a + b)
(2a + b) : (3a + b)
Solution
ABCD is a trapezium with parallel sides AB = a cm and DC = b cm (Figure). E and F are the mid-points of the non-parallel sides. The ratio of ar (ABFE) and ar (EFCD) is (3a + b) : (a + 3b).
Explanation:
Given, AB = a cm, DC = b cm and AB || DC.
Also, E and F are mid-points of AD and BC, respectively.
So, distance between CD, EF and AB, EF will be same say h.
Join BD which intersect EF at M.
Now, in ΔABD, E is the mid-point of AD and EM || AB
So, M is the mid-point of BD
And EM = `1/2`AB [By mid-point theorem] ...(i)
Similarly in ΔCBD, MF = `1/2`CD ...(ii)
On adding equations (i) and (ii), we get
EM + MF = `1/2` AB + `1/2` CD
⇒ EF = `1/2`(AB + CD) = `1/2`(a + b)
Now, area of trapezium ABFE
= `1/2`(sum of parallel sides) × (distance between parallel sides)
= `1/2(a + 1/2(a + b)) xx h`
= `1/4(3a + b)h`
Now, area of trapezium EFCD
= `1/2[b + 1/2(a + b)] xx h`
= `1/4(3b + a)h`
∴ Required ratio = `"Area of ABFE"/"Area of EFCD"`
= `(1/4(3a + b)h)/(1/4(3b + a)h)`
= `((3a + b))/((a + 3b))` or (3a + b) : (a + 3b)
APPEARS IN
RELATED QUESTIONS
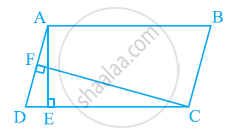
In the given figure, ABCD is parallelogram, AE ⊥ DC and CF ⊥ AD. If AB = 16 cm, AE = 8 cm and CF = 10 cm, find AD.
P and Q are any two points lying on the sides DC and AD respectively of a parallelogram ABCD. Show that ar (APB) = ar (BQC).
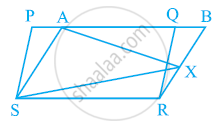
In the given figure, PQRS and ABRS are parallelograms and X is any point on side BR. Show that
(i) ar (PQRS) = ar (ABRS)
(ii) ar (AXS) = 1/2ar (PQRS)
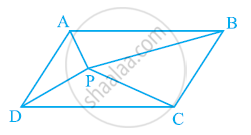
In the given figure, P is a point in the interior of a parallelogram ABCD. Show that
(i) ar (APB) + ar (PCD) = 1/2ar (ABCD)
(ii) ar (APD) + ar (PBC) = ar (APB) + ar (PCD)
[Hint: Through. P, draw a line parallel to AB]
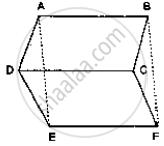
In the following figure, ABCD, DCFE and ABFE are parallelograms. Show that ar (ADE) = ar (BCF).
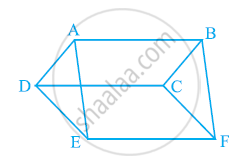
In the given below fig. ABCD, ABFE and CDEF are parallelograms. Prove that ar (ΔADE)
= ar (ΔBCF)
ABCD is a parallelogram, G is the point on AB such that AG = 2 GB, E is a point of DC
such that CE = 2DE and F is the point of BC such that BF = 2FC. Prove that:
(1) ar ( ADEG) = ar (GBCD)
(2) ar (ΔEGB) = `1/6` ar (ABCD)
(3) ar (ΔEFC) = `1/2` ar (ΔEBF)
(4) ar (ΔEBG) = ar (ΔEFC)
(5)ΔFind what portion of the area of parallelogram is the area of EFG.
In the following figure, PSDA is a parallelogram. Points Q and R are taken on PS such that PQ = QR = RS and PA || QB || RC. Prove that ar (PQE) = ar (CFD).

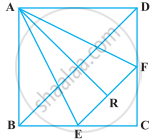
ABCD is a square. E and F are respectively the mid-points of BC and CD. If R is the mid-point of EF (Figure), prove that ar (AER) = ar (AFR)
The diagonals of a parallelogram ABCD intersect at a point O. Through O, a line is drawn to intersect AD at P and BC at Q. Show that PQ divides the parallelogram into two parts of equal area.
