Advertisements
Advertisements
Question
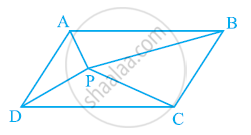
In the given figure, P is a point in the interior of a parallelogram ABCD. Show that
(i) ar (APB) + ar (PCD) = 1/2ar (ABCD)
(ii) ar (APD) + ar (PBC) = ar (APB) + ar (PCD)
[Hint: Through. P, draw a line parallel to AB]
Advertisements
Solution
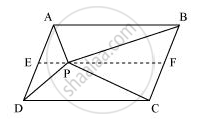
(i) Let us draw a line segment EF, passing through point P and parallel to line segment AB.
In parallelogram ABCD,
AB || EF (By construction) ... (1)
ABCD is a parallelogram.
∴ AD || BC (Opposite sides of a parallelogram)
⇒ AE || BF ... (2)
From equations (1) and (2), we obtain
AB || EF and AE || BF
Therefore, quadrilateral ABFE is a parallelogram.
It can be observed that ΔAPB and parallelogram ABFE are lying on the same base AB and between the same parallel lines AB and EF.
∴ Area (ΔAPB) = 1/2Area (ABFE) ... (3)
Similarly, for ΔPCD and parallelogram EFCD,
Area (ΔPCD) = 1/2Area (EFCD) ... (4)
Adding equations (3) and (4), we obtain
Area (ΔAPB) + Area (ΔPCD) = 1/2[Area (ABFE) + Area (EFCD)]
Area (ΔAPB) + Area (ΔPCD) = 1/2Area (ABCD) ........(5)
(ii)
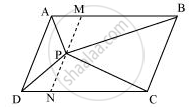
Let us draw a line segment MN, passing through point P and parallel to line segment AD.
In parallelogram ABCD,
MN || AD (By construction) ... (6)
ABCD is a parallelogram.
∴ AB || DC (Opposite sides of a parallelogram)
⇒ AM || DN ... (7)
From equations (6) and (7), we obtain
MN || AD and AM || DN
Therefore, quadrilateral AMND is a parallelogram.
It can be observed that ΔAPD and parallelogram AMND are lying on the same base AD and between the same parallel lines AD and MN.
∴ Area (ΔAPD) = 1/2Area (AMND) ... (8)
Similarly, for ΔPCB and parallelogram MNCB,
Area (ΔPCB) = 1/2Area (MNCB) ... (9)
Adding equations (8) and (9), we obtain
Area (ΔAPD) + Area (ΔPCB) = 1/2[Area (AMND) + Area (MNCB)]
Area (ΔAPD) + Area (ΔPCB) = 1/2Area (ABCD) ...........(10)
On comparing equations (5) and (10), we obtain
Area (ΔAPD) + Area (ΔPBC) = Area (ΔAPB) + Area (ΔPCD)
APPEARS IN
RELATED QUESTIONS
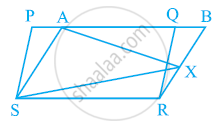
In the given figure, ABCD is parallelogram, AE ⊥ DC and CF ⊥ AD. If AB = 16 cm, AE = 8 cm and CF = 10 cm, find AD.
In the given below fig. ABCD, ABFE and CDEF are parallelograms. Prove that ar (ΔADE)
= ar (ΔBCF)
ABCD is a parallelogram, G is the point on AB such that AG = 2 GB, E is a point of DC
such that CE = 2DE and F is the point of BC such that BF = 2FC. Prove that:
(1) ar ( ADEG) = ar (GBCD)
(2) ar (ΔEGB) = `1/6` ar (ABCD)
(3) ar (ΔEFC) = `1/2` ar (ΔEBF)
(4) ar (ΔEBG) = ar (ΔEFC)
(5)ΔFind what portion of the area of parallelogram is the area of EFG.
In the below fig. ABCD and AEFD are two parallelograms. Prove that
(1) PE = FQ
(2) ar (Δ APE) : ar (ΔPFA) = ar Δ(QFD) : ar (Δ PFD)
(3) ar (ΔPEA) = ar (ΔQFD)
In which of the following figures, you find two polygons on the same base and between the same parallels?
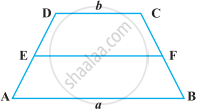
ABCD is a trapezium with parallel sides AB = a cm and DC = b cm (Figure). E and F are the mid-points of the non-parallel sides. The ratio of ar (ABFE) and ar (EFCD) is ______.
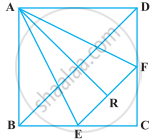
ABCD is a square. E and F are respectively the mid-points of BC and CD. If R is the mid-point of EF (Figure), prove that ar (AER) = ar (AFR)
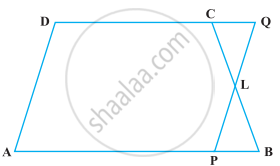
In trapezium ABCD, AB || DC and L is the mid-point of BC. Through L, a line PQ || AD has been drawn which meets AB in P and DC produced in Q (Figure). Prove that ar (ABCD) = ar (APQD)
The diagonals of a parallelogram ABCD intersect at a point O. Through O, a line is drawn to intersect AD at P and BC at Q. Show that PQ divides the parallelogram into two parts of equal area.
In the following figure, ABCD and AEFD are two parallelograms. Prove that ar (PEA) = ar (QFD). [Hint: Join PD].
