Advertisements
Advertisements
Question
ABCD is a trapezium in which AB || DC, DC = 30 cm and AB = 50 cm. If X and Y are, respectively the mid-points of AD and BC, prove that ar (DCYX) = `7/9` ar (XYBA)
Solution
Given: In a trapezium ABCD, AB || DC, DC = 30 cm and AB = 50 cm.
Also, X and Y are respectively the mid-points of AD and BC.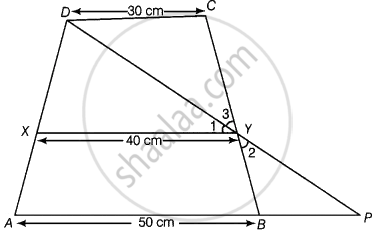
To prove: `ar (DCYX) = 7/9 ar (XYBA)`
Construction: Join DY and extend it to meet produced AB at P.
Proof: In ΔDCY and ΔPBY,
CY = BY ...[Since, Y is the mid-point of BC]
∠DCY = ∠PBY ...[Alternate interior angles]
And ∠2 = ∠3 ...[Vertically opposite angles]
∴ ΔDCY ≅ ΔPBY ...[By ASA congruence rule]
Then, DC = BP ...[By CPCT]
But DC = 30 cm ...[Given]
∴ DC = BP = 30 cm
Now, AP = AB + BP
= 50 + 30
= 80 cm
In ΔADP, by mid-point theorem,
`XY = 1/2 AP`
= `1/2 xx 80`
= 40 cm
Let distance between AB, XY and XY, DC is h cm.
Now, area of trapezium `DCYX = 1/2 h (30 + 40)` ...[∵ Area of trapezium = `1/2` sum of parallel sides × distance between them]
= `1/2 h (70)`
= 35 h cm2
Similarly, area of trapezium XYBA
= `1/2 h (40 + 50)`
= `1/2 h xx 90`
= 45 h cm2
∴ `(ar (DCYX))/(ar (XYBA)) = (35h)/(45h) = 7/9`
⇒ `ar (DCYX) = 7/9 ar (XYBA)`
Hence proved.
APPEARS IN
RELATED QUESTIONS
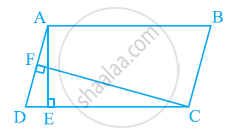
In the given figure, ABCD is parallelogram, AE ⊥ DC and CF ⊥ AD. If AB = 16 cm, AE = 8 cm and CF = 10 cm, find AD.
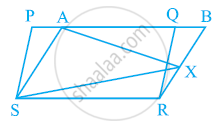
In the given figure, PQRS and ABRS are parallelograms and X is any point on side BR. Show that
(i) ar (PQRS) = ar (ABRS)
(ii) ar (AXS) = 1/2ar (PQRS)
Parallelogram ABCD and rectangle ABEF are on the same base AB and have equal areas. Show that the perimeter of the parallelogram is greater than that of the rectangle.
ABCD is a parallelogram, G is the point on AB such that AG = 2 GB, E is a point of DC
such that CE = 2DE and F is the point of BC such that BF = 2FC. Prove that:
(1) ar ( ADEG) = ar (GBCD)
(2) ar (ΔEGB) = `1/6` ar (ABCD)
(3) ar (ΔEFC) = `1/2` ar (ΔEBF)
(4) ar (ΔEBG) = ar (ΔEFC)
(5)ΔFind what portion of the area of parallelogram is the area of EFG.
In which of the following figures, you find two polygons on the same base and between the same parallels?
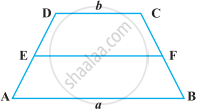
ABCD is a trapezium with parallel sides AB = a cm and DC = b cm (Figure). E and F are the mid-points of the non-parallel sides. The ratio of ar (ABFE) and ar (EFCD) is ______.
In the following figure, PSDA is a parallelogram. Points Q and R are taken on PS such that PQ = QR = RS and PA || QB || RC. Prove that ar (PQE) = ar (CFD).

ABCD is a parallelogram in which BC is produced to E such that CE = BC (Figure). AE intersects CD at F. If ar (DFB) = 3 cm2, find the area of the parallelogram ABCD.
The diagonals of a parallelogram ABCD intersect at a point O. Through O, a line is drawn to intersect AD at P and BC at Q. Show that PQ divides the parallelogram into two parts of equal area.
In the following figure, ABCD and AEFD are two parallelograms. Prove that ar (PEA) = ar (QFD). [Hint: Join PD].

