Advertisements
Advertisements
Question
Solve the following quadratic equation by completing the square method.
m2 – 5m = –3
Solution
m2 – 5m = –3
\[\Rightarrow m^2 - 5m + \left( \frac{- 5}{2} \right)^2 - \left( \frac{- 5}{2} \right)^2 = - 3\]
\[ \Rightarrow \left( m^2 - 5m + \frac{25}{4} \right) - \frac{25}{4} = - 3\]
\[ \Rightarrow \left( m - \frac{5}{2} \right)^2 = - 3 + \frac{25}{4}\]
\[ \Rightarrow \left( m - \frac{5}{2} \right)^2 = \frac{13}{4}\]
\[ \Rightarrow \left( m - \frac{5}{2} \right)^2 = \left( \frac{\sqrt{13}}{2} \right)^2 \]
\[ \Rightarrow m - \frac{5}{2} = \frac{\sqrt{13}}{2} \text{ or } m - \frac{5}{2} = - \frac{\sqrt{13}}{2}\]
\[ \Rightarrow m = \frac{\sqrt{13}}{2} + \frac{5}{2} \text{ or } m = - \frac{\sqrt{13}}{2} + \frac{5}{2}\]
\[ \Rightarrow m = \frac{\sqrt{13} + 5}{2} \text{ or } m = \frac{5 - \sqrt{13}}{2}\]
APPEARS IN
RELATED QUESTIONS
The sum of the reciprocals of Rehman's ages, (in years) 3 years ago and 5 years from now is 1/3. Find his present age.
The diagonal of a rectangular field is 60 metres more than the shorter side. If the longer side is 30 metres more than the shorter side, find the sides of the field.
Find the roots of the following quadratic equations (if they exist) by the method of completing the square.
`sqrt3x^2+10x+7sqrt3=0`
`sqrt3x^2+10x+7sqrt3=0`
A farmer prepares rectangular vegetable garden of area 180 sq meters. With 39 meters of barbed wire, he can fence the three sides of the garden, leaving one of the longer sides unfenced. Find the dimensions of the garden.
The area of right -angled triangle is 165 sq meters. Determine its base and altitude if the latter exceeds the former by 7 meters.
The hypotenuse of a right=-angled triangle is 20 meters. If the difference between the lengths of the other sides be 4 meters, find the other sides
The length of the hypotenuse of a right-angled triangle exceeds the length of the base by 2 cm and exceeds twice the length of the altitude by 1 cm. Find the length of each side of the triangle.
Solve the following quadratic equation by completing the square method.
9y2 – 12y + 2 = 0
Solve the following quadratic equation by completing the square method.
5x2 = 4x + 7
Fill in the gaps and complete.
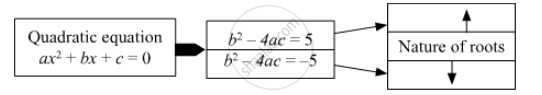
Fill in the gap and complete.

Determine the nature of roots of the following quadratic equation.
m2 + 2m + 9 = 0
The sum of the squares of two consecutive even numbers is 340. Find the numbers.
The value of \[\sqrt{6 + \sqrt{6 + \sqrt{6 +}}} . . . .\] is
For solving the quadratic equations `"x"^2 + 8"x" =-15` by completing the square method, find the third term.
The sum of the areas of two squares is 400 sq.m. If the difference between their perimeters is 16 m, find the sides of two squares.
One year ago, a man was 8 times as old as his son. Now his age is equal to the square of his son’s age. Their present ages are:
Rohini had scored 10 more marks in her mathematics test out of 30 marks, 9 times these marks would have been the square of her actual marks. How many marks did she get on the test?
