Advertisements
Advertisements
Question
Solve the inequation:
`– 1 < 3 – "x"/5 ≤ 1`
Solution
`– 1 < 3 – "x"/5 ≤ 1`
Multiplying the inequation by 5, the sign of inequality changes
5 > – 15 + x > – 5
Adding 15 on both the sides, we get
20 > x > 10
⇒ 10 < x < 20
The solution contains all the real values of x lying between 10 and 20, excluding the boundary value.
The solution set can be written in the form of open interval (10, 20).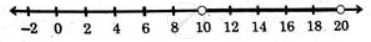
APPEARS IN
RELATED QUESTIONS
Solve the following inequation: 3x – 36 > 0
Solve the following inequation:
7x – 25 ≤ – 4
Solve the following inequation: |7x – 4| < 10
Solve the inequation:
3x + 1 ≥ 6x – 4
Solve the inequation:
4 – 2x < 3(3 – x)
Solve the inequation:
`3/4 "x" - 6 ≤ "x" - 7`
Solve the inequation:
– 8 ≤ – (3x – 5) < 13
Solve the inequation:
2|4 – 5x| ≥ 9
Solve the inequation:
`("x" + 5)/("x" - 3) < 0`
The region represented by {z = x + iy ∈ C : |z| – Re(z) ≤ 1} is also given by the inequality is ______.
Consider the two sets : A = {m ∈ R : both the roots of x2 – (m + 1)x + m + 4 = 0 are real} and B = [–3, 5). Which of the following is not true?
Let S be the set of all real roots of the equation, 3x(3x – 1) + 2 = |3x – 1| + |3x – 2|. Then S ______.
Let Z be the set of integers. lf A = `{x ∈ Z: 2^((x + 2)(x^2 – 5x + 6)) = 1}` and B = {x ∈ Z: –3 < 2x – 1 < 9}, then the number of subsets of the set A × B, is ______.
