Advertisements
Advertisements
Question
State the law of conservation of angular momentum and explain with a suitable example.
Solution
Statement: The angular momentum of a body remains constant if the resultant external torque acting on the body is zero.
Example:
- A ballet dancer makes use of the law of conservation of angular momentum to vary her angular speed.
- The torque acting on her body is zero. By the law of conservation of angular momentum, L = constant
Iω = constant ⇒ ω ∝1/I - When she suddenly folds her arms and brings the stretched leg close to the body, her angular velocity increases on account of the decrease in moment of inertia. This helps in rotating safely on her legs.
APPEARS IN
RELATED QUESTIONS
Obtain an expression for torque acting on a body rotating with uniform angular acceleration.
Define moment of inertia. State its SI unit and dimensions.
A stone is tied to one end of a string. Holding the other end, the string is whirled in a horizontal plane with progressively increasing speed. It breaks at some speed because ______
A flywheel of mass 8 kg and radius 10 cm rotating with a uniform angular speed of 5 rad/sec about its axis of rotation, is subjected to an accelerating torque of 0.01 Nm for 10 seconds. Calculate the change in its angular momentum and change in its kinetic energy.
An electron(e) is revolving in a circular orbit of radius r in the hydrogen atom. The angular momentum of the electron is (M = magnetic dipole moment associated with it and m = mass of electron)
A charged particle (charge = q: mass = m) is rotating in a circle of radius 'R' with uniform speed 'v'. The ratio of its magnetic moment (M) to the angular momentum (L) is ______
If the angular momentum of a body increases by 50%, then its kinetic energy of rotation increases by ______ (M.I. remains constant)
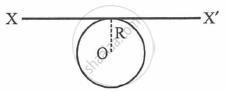
A thin metal wire of length 'L' and uniform linear mass density 'ρ' is bent into a circular coil with 'O' as centre. The moment of inertia of a coil about the axis XX' is ______.
If the kinetic energy of rotation of a body is doubled, then its angular momentum ____________.
Two bodies with moments of inertia I1 and I2 (I1 > I2) have equal angular momenta. lf E1 and E2 are their rotational kinetic energies respectively, then ____________.
A particle of mass m is rotating in a plane in a circular path of radius r. Its angular momentum is L. The centripetal force acting on the particle is ______.
If the angular momentum of an electron is `vec"J"` then the magnitude of the magnetic moment will be ____________.
Earth revolves round the sun in a circular orbit of radius 'R'. The angular momentum of the revolving earth is directly proprtional to ______.
An electron has a mass of 9.1 x 10-31 kg. It revolves round the nucleus in a circular orbit of radius 0.529 x 10-10 metre at a speed of 2.2 x 106 m/s. The magnitude of its linear momentum in this motion is ____________.
An electron of mass 'm' revolving around the nucleus in a circular orbit of radius 'r' has angular momentum 'L'. The magnetic field produced by the electron at the centre of the orbit is e = electric charge, µ0 = permeability of free space ____________.
A particle is revolving in anticlockwise sense along the circumference of a circle of radius 'r' with linear velocity 'v', then the angle between 'v' and angular velocity 'ω' will be ______.
Three-point masses each of mass 'M' are placed at the corners of an equilateral triangle of side 'a'. The moment of inertia of this system about an axis passing through one side of a triangle is ______.
An electron in an atom is revolving round the nucleus in a circular orbit of radius 5.3 × 10-11 m with a speed of 3 × 106 m/s. Find the angular momentum of electron.
A particle of mass m = 5 unit is moving with a uniform speed v = 3`sqrt2` unit in the XY-plane along the line y = x + 4. The magnitude of the angular momentum about origin is ______.
The difference in the angular momentum of an electron in two successive orbits of a hydrogen atom is ______.
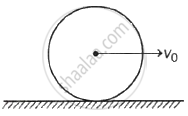
A sphere rolls without slipping on a rough horizontal surface with centre of mass speed v0. If mass of the sphere is M and its radius is R, then what is the angular momentum of the sphere about the point of contact?
The angular momentum of the electron in the second orbit of hydrogen atom is L. The angular momentum in the third orbit is ______.
Define angular momentum.
Calculate the change in angular momentum of the electron when it jumps from third orbit to first orbit in hydrogen atom.
(Take h = 6.33 × 10−34 Js)
