Advertisements
Advertisements
Question
Students of a school are standing in rows and columns in their playground for a drill practice. A, B, C and D are the positions of four students as shown in figure. Is it possible to place Jaspal in the drill in such a way that he is equidistant from each of the four students A, B, C and D? If so, what should be his position?
Solution
Yes, from the figure we observe that the positions of four students A, B, C and D are (3, 5), (7, 9), (11, 5) and (7, 1) respectively i.e., these are four vertices of a quadrilateral.
Now, we will find the type of this quadrilateral.
For this, we will find all its sides.
We see that, AB = BC = CD = DA i.e., all sides are equal.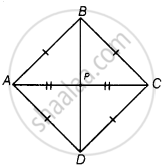
Now, AB = `sqrt((7 - 3)^2 + (9 - 5)^2` ...`["By distance formula", d = sqrt((x_2 - x_1)^2 + (y_2 - y_1)^2)]`
AB = `sqrt((4)^2 + (4)^2`
= `sqrt(16 + 16)`
AB = `4sqrt(2)`
BC = `sqrt((11 - 7)^2 + (5 - 9)^2`
= `sqrt((4)^2 + (-4)^2`
= `sqrt(16 + 16)`
= `4sqrt(2)`
CD = `sqrt((7 - 11)^2 + (1 - 5)^2`
= `sqrt((-4)^2 + (-4)^2`
= `sqrt(16 + 16)`
= `4sqrt(2)`
And DA = `sqrt((3 - 7)^2 + (5 - 1)^2`
= `sqrt((-4)^2 + (4)^2`
= `sqrt(16 + 16)`
= `4sqrt(2)`
We see that, AB = BC = CD = DA i.e., all sides are equal.
Now, we find length of both diagonals.
AC = `sqrt((11 - 3)^2 + (5 - 5)^2`
= `sqrt((8)^2 + 0)`
= 8
And BD = `sqrt((7 - 7)^2 + (1 - 9)^2`
= `sqrt(0 + (-8)^2`
= 8
Here, AC = BD
Since, AB = BC = CD = DA and AC = BD
Which represent a square.
Also known the diagonals of a square bisect each other.
So, P be position of Jaspal in which he is equidistant from each of the four students A, B, C and D.
∴ Coordinates of point P = Mid-point of AC
= `((3 + 11)/2, (5 + 5)/2)` ...`[∵ "Since, mid-point of a line segment having points" (x_1, y_1) "and" (x_2, y_2) = ((x_1 + y_1)/2, (x_2 + y_2)/2)]`
= `(14/2, 10/2)`
= (7, 5)
Hence, the required position of Jaspal is (7, 5).
APPEARS IN
RELATED QUESTIONS
A line intersects the y-axis and x-axis at the points P and Q respectively. If (2, –5) is the mid-point of PQ, then find the coordinates of P and Q.
On which axis do the following points lie?
P(5, 0)
Two vertices of an isosceles triangle are (2, 0) and (2, 5). Find the third vertex if the length of the equal sides is 3.
Find the value of k, if the point P (0, 2) is equidistant from (3, k) and (k, 5).
Find a point on the x-axis which is equidistant from the points (7, 6) and (−3, 4).
Prove that the points (3, 0), (4, 5), (-1, 4) and (-2, -1), taken in order, form a rhombus.
Also, find its area.
Find the coordinates of the point where the diagonals of the parallelogram formed by joining the points (-2, -1), (1, 0), (4, 3) and(1, 2) meet
Prove that the points (4, 5) (7, 6), (6, 3) (3, 2) are the vertices of a parallelogram. Is it a rectangle.
Show that the following points are the vertices of a square:
(i) A (3,2), B(0,5), C(-3,2) and D(0,-1)
Find the ratio in which the line segment joining the points A(3, −3) and B(−2, 7) is divided by the x-axis. Also, find the coordinates of the point of division.
If A(−3, 5), B(−2, −7), C(1, −8) and D(6, 3) are the vertices of a quadrilateral ABCD, find its area.
Write the distance between the points A (10 cos θ, 0) and B (0, 10 sin θ).
What is the area of the triangle formed by the points O (0, 0), A (6, 0) and B (0, 4)?
If points Q and reflections of point P (−3, 4) in X and Y axes respectively, what is QR?
Write the formula for the area of the triangle having its vertices at (x1, y1), (x2, y2) and (x3, y3).
Find the area of triangle with vertices ( a, b+c) , (b, c+a) and (c, a+b).
The line segment joining points (−3, −4), and (1, −2) is divided by y-axis in the ratio.
The ratio in which the line segment joining P (x1, y1) and Q (x2, y2) is divided by x-axis is
Ordinate of all points on the x-axis is ______.
In which quadrant, does the abscissa, and ordinate of a point have the same sign?
