Advertisements
Advertisements
Question
The angles of elevation and depression of the top and the bottom of a tower from the top of a building, 60 m high, are 30° and 60° respectively. Find the difference between the heights of the building and the tower and the distance between them.
Solution
Let AB be the building and CD be the tower.
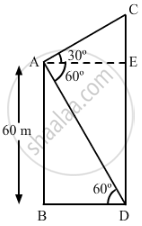
In right ∆ABD:
`(AB)/(BD)=tan 60^@`
`⇒ 60/(BD)=sqrt3`
`⇒ BD=60/sqrt3`
`⇒ BD=20sqrt3`
In right ∆ACE:
`(CE)/(AE)=tan 30^@`
`⇒ (CE)/(BD)=1/sqrt3 (∵AE=BD)`
`⇒CE=(20sqrt3)/sqrt3=20`
Height of the tower = CE + ED = CE + AB = 20 m + 60 m = 80 m
Difference between the heights of the tower and the building = 80 m − 60 m = 20 m
Distance between the tower and the building = BD = `20sqrt3`
APPEARS IN
RELATED QUESTIONS
When the sun is 30º above the horizontal, Find the length of shadow cast by a building 50m high
The heights of two poles are 80 m and 62.5 m. If the line joining their tops makes an angle of 45º with the horizontal, then find the distance between the pole
The angle of elevation of the top of a building from the foot of the tower is 30° and the angle of elevation of the top of the tower from the foot of the building is 60°. If the tower is 50 m high, find the height of the building.
From the top of the light house, an observer looks at a ship and finds the angle of depression to be 30°. If the height of the light-house is 100 meters, then find how far the ship is from the light-house.
A ladder makes an angle of 60º with the ground when placed against a wall. If the foot of the ladder is 2 m away from the wall, then the length of the ladder (in metres) is
A tower is 60 m heigh. Its shadow is x metres shorter when the sun’s altitude is 45° than when it has been 30°, then x is equal to
A portion of a 60 m long tree is broken by a tornado and the top struck up the ground making an angle of 30° with the ground level. The height of the point where the tree is broken is equal to ____________.
The angles of depression of two objects from the top of a 100 m hill lying to its east are found to be 45° and 30°. Find the distance between the two objects. (Take `sqrt3 = 1.73`)
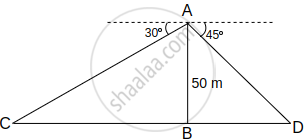
In the given figure, AB is tower of height 50 m. A man standing on its top, observes two car on the opposite sides of the tower with angles of depression 30° and 45° respectively. Find the distance between the two cars.
The top of a hill when observed from the top and bottom of a building of height h is at angles of elevation p and q respectively. What is the height of the hill?
