Advertisements
Advertisements
Question
In the given figure, AB is tower of height 50 m. A man standing on its top, observes two car on the opposite sides of the tower with angles of depression 30° and 45° respectively. Find the distance between the two cars.
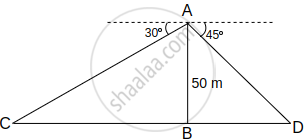
Solution

Clearly ∠ADB = 45° and ∠ACB = 30° ....[∵ Angle of depression = Angle of elevation]
Now, In ΔABD, we have
tan 45° = `(AB)/(BD)`
⇒ 1 = `50/(BD)`
⇒ BD = 50 m ...(i)
Also, In ΔABC, we have
tan 30° = `(AB)/(BC)`
⇒ `1/sqrt(3) = (AB)/(BC)`
⇒ BC = `ABsqrt(3) = 50sqrt(3)` m ...(ii)
From equations (i) and (ii), we get
CD = BC + BD
= `(50sqrt(3) + 50)`m
= `50(sqrt(3) + 1)`m
= 50(1.732 + 1)
= 50 × 2.732
= 136.6 m
Thus, the distance between two cars is 136.6 m.
RELATED QUESTIONS
The angle of elevation of an aeroplane from point A on the ground is 60˚. After flight of 15 seconds, the angle of elevation changes to 30˚. If the aeroplane is flying at a constant height of 1500√3 m, find the speed of the plane in km/hr.
From a point on the ground, the angles of elevation of the bottom and the top of a transmission tower fixed at the top of a 20 m high building are 45° and 60° respectively. Find the height of the tower. (Use `sqrt3` = 1.73)
A statue, 1.6 m tall, stands on a top of pedestal, from a point on the ground, the angle of elevation of the top of statue is 60° and from the same point the angle of elevation of the top of the pedestal is 45°. Find the height of the pedestal.
A tower stands vertically on the ground. From a point on the ground 20 m away from the foot of the tower, the angle of elevation of the top of the tower is 60°. What is the height of the tower?
From the top of a cliff 25 m high the angle of elevation of a tower is found to be equal to the angle of depression of the foot of the tower. The height of the tower is
The angle of elevation of a cloud from a point h metre above a lake is θ. The angle of depression of its reflection in the lake is 45°. The height of the cloud is
From the top of the tower 60 m high the angles of depression of the top and bottom of a vertical lamp post are observed to be 38° and 60° respectively. Find the height of the lamp post (tan 38° = 0.7813, `sqrt(3)` = 1.732)
A bird is sitting on the top of a 80 m high tree. From a point on the ground, the angle of elevation of the bird is 45°. The bird flies away horizontally in such away that it remained at a constant height from the ground. After 2 seconds, the angle of elevation of the bird from the same point is 30°. Determine the speed at which the bird flies `(sqrt(3) = 1.732)`
The angle of elevation of the top of a tower from a point 20 meters away from its base is 45°. The height of the tower is ____________.
The angle of elevation of the top of a tower 30 m high from the foot of another tower in the same plane is 60° and the angle of elevation of the top of the second tower from the foot of the first tower is 30°. Find the distance between the two towers and also the height of the other tower.
