Advertisements
Advertisements
Question
The angles of a quadrilateral are in the ratio 2 : 4 : 5 : 7. Find all the angles
Solution
Let the angles of a quadrilateral be 2x, 4x, 5x, and 7x.
Total angle of a quadrilateral = 360°
2x + 4x + 5x + 7x = 360°
18° = 360°
x = `(360^circ)/18`
= 20°
2x = 2 × 20° = 40°, 4x = 4 × 20° = 80°,
5x = 5 × 20° = 100°, 7x = 7 × 20° = 140°
The angles of a quadrilateral are 40°, 80°, 100° and 140°.
APPEARS IN
RELATED QUESTIONS
Complete of the following, so as to make a true statement:
A quadrilateral has .... diagonals.
In Fig. 16.19, ABCD is a quadrilateral.
Name a pair of adjacent angles.
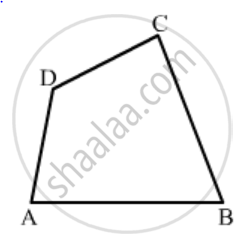
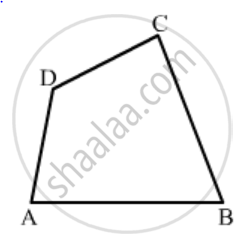
In Fig. 16.19, ABCD is a quadrilateral.
How many pairs of adjacent angles are there?
In a quadrilateral ABCD, CO and DO are the bisectors of ∠C and ∠D respectively. Prove that \[∠COD = \frac{1}{2}(∠A + ∠B) .\]
Complete the following statement by means of one of those given in brackets against each:
If one pair of opposite sides are equal and parallel, then the figure is ........................
Complete the following statement by means of one of those given in brackets against each:
f consecutive sides of a parallelogram are equal, then it is necessarily a ..................
Two adjacent angles of a parallelogram are 70° and 110° respectively. Find the other two angles of it.
The number of common points in the two angles marked in figure is ______.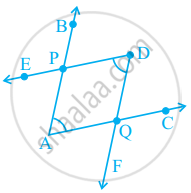
In given figure, What is AE + EC?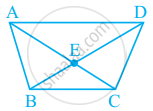
What conclusion can be drawn from part of given figure, if BD bisects ∠ABC?
