Advertisements
Advertisements
Question
The areas of two similar triangles are 169 cm2 and 121 cm2 respectively. If the longest side of the larger triangle is 26 cm, find the longest side of the smaller triangle.
Solution
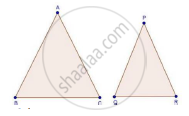
We have,
ΔABC ~ ΔPQR
Area(ΔABC) = 169 cm2
Area(ΔPQR) = 121 cm2
And AB = 26 cm
By area of similar triangle theorem
`("Area"(triangleABC))/("Area"(trianglePQR))="AB"^2/"PQ"^2`
`rArr169/121=26^2/"PQ"^2`
`rArr13/11=26/"PQ"` [Taking square root]
`rArr"PQ"=11/13xx26=22` cm
APPEARS IN
RELATED QUESTIONS
In two similar triangles ABC and PQR, if their corresponding altitudes AD and PS are in the ratio 4 : 9, find the ratio of the areas of ∆ABC and ∆PQR
D and E are points on the sides AB and AC respectively of a ∆ABC such that DE || BC and divides ∆ABC into two parts, equal in area. Find
Two isosceles triangles have equal vertical angles and their areas are in the ratio 16 : 25. Find the ratio of their corresponding heights
The areas of two similar triangles are 81 cm2 and 49 cm2 respectively. Find the ratio of their corresponding heights. What is the ratio of their corresponding medians?
The areas of two similar triangles are 121 cm2 and 64 cm2 respectively. If the median of the first triangle is 12.1 cm, find the corresponding median of the other.
Areas of two similar triangles are 225 sq.cm. 81 sq.cm. If a side of the smaller triangle is 12 cm, then Find corresponding side of the bigger triangle.
If ∆XYZ ~ ∆PQR and A(∆XYZ) = 25 cm2, A(∆PQR) = 4 cm2 then XY : PQ = ?
Ratio of areas of two similar triangles is 9 : 25. _______ is the ratio of their corresponding sides.
O is a point on side PQ of a APQR such that PO = QO = RO, then ______.
Use area theorem of similar triangles to prove congruency of two similar triangles with equal areas.
