Advertisements
Advertisements
Question
The areas of two similar triangles are 25 cm2 and 36 cm2 respectively. If the altitude of the first triangle is 2.4 cm, find the corresponding altitude of the other.
Solution
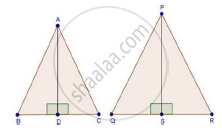
We have,
ΔABC ~ ΔPQR
Area (ΔABC) = 25 cm2
Area (ΔPQR) = 36 cm2
AD = 2.4 cm
And AD and PS are the altitudes
To find: PS
Proof: Since, ΔABC ~ ΔPQR
Then, by area of similar triangle theorem
`("Area"(triangleABC))/("Area"(trianglePQR))="AB"^2/"PQ"^2`
`rArr25/36="AB"^2/"PQ"^2`
`rArr5/6="AB"/"PQ"` .........(i)
In ΔABD and ΔPQS
∠B = ∠Q [Δ ABC ~ ΔPQR]
∠ADB ~ ∠PSQ [Each 90°]
Then, ΔABD ~ ΔPQS [By AA similarity]
`therefore"AB"/"PS"="AD"/"PS"` ......(ii) [Corresponding parts of similar Δ are proportional]
Compare (i) and (ii)
`"AD"/"PS"=5/6`
`rArr2.4/"PS"=5/6`
`"PS"=(2.4xx6)/5=2.88` cm
APPEARS IN
RELATED QUESTIONS
Prove that the area of the triangle BCE described on one side BC of a square ABCD as base is one half the area of the similar Triangle ACF described on the diagonal AC as base
If the areas of two similar triangles are equal, prove that they are congruent.
Sides of two similar triangles are in the ratio 4 : 9. Areas of these triangles are in the ratio
In ΔABC, PQ is a line segment intersecting AB at P and AC at Q such that PQ || BC and PQ divides ΔABC into two parts equal in area. Find `(BP)/(AB)`
ABC is a triangle and PQ is a straight line meeting AB in P and AC in Q. If AP = 1 cm, PB = 3 cm, AQ = 1.5 cm, QC = 4.5 m, prove that area of ΔAPQ is one- sixteenth of the area of ABC.
If ∆ABC ~ ∆PQR, A (∆ABC) = 80, A (∆PQR) = 125, then fill in the blanks. \[\frac{A\left( ∆ ABC \right)}{A\left( ∆ . . . . \right)} = \frac{80}{125} \therefore \frac{AB}{PQ} = \frac{......}{......}\]
∆LMN ~ ∆PQR, 9 × A (∆PQR ) = 16 × A (∆LMN). If QR = 20 then Find MN.
∆MNT ~ ∆QRS. Length of altitude drawn from point T is 5 and length of altitude drawn from point S is 9. Find the ratio `("A"(Δ"MNT"))/("A"(Δ"QRS"))`.
In ΔLMN, ∠L = 50° and ∠N = 60°, If ΔLMN ~ ΔPQR, then find ∠Q.
In the adjoining figure, ΔADB ∼ ΔBDC. Prove that BD2 = AD × DC.