Advertisements
Advertisements
Question
∆LMN ~ ∆PQR, 9 × A (∆PQR ) = 16 × A (∆LMN). If QR = 20 then Find MN.
Solution
Given:
QR = 20
∆LMN ~ ∆PQR
9 × A (∆PQR ) = 16 × A (∆LMN)
Consider, 9 × A (∆PQR ) = 16 × A (∆LMN)
\[\frac{A\left( ∆ LMN \right)}{A\left( ∆ PQR \right)} = \frac{9}{16}\]
\[ \Rightarrow \frac{{MN}^2}{{QR}^2} = \frac{3^2}{4^2}\]
\[ \Rightarrow \frac{MN}{QR} = \frac{3}{4}\]
\[\Rightarrow MN = \frac{3}{4} \times QR\]
\[ \Rightarrow MN = \frac{3}{4} \times 20 \left[ \because QR = 20 \right]\]
\[ \Rightarrow MN = 15\]
APPEARS IN
RELATED QUESTIONS
In two similar triangles ABC and PQR, if their corresponding altitudes AD and PS are in the ratio 4 : 9, find the ratio of the areas of ∆ABC and ∆PQR
D, E, F are the mid-point of the sides BC, CA and AB respectively of a ∆ABC. Determine the ratio of the areas of ∆DEF and ∆ABC.
In Figure, ABC and DBC are two triangles on the same base BC. If AD intersects BC at O, show that `(ar(ABC))/(ar(DBC)) = (AO)/(DO)`
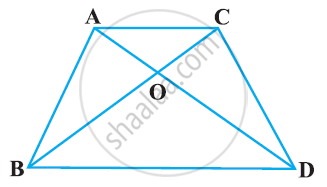
If the areas of two similar triangles are equal, prove that they are congruent.
D, E and F are respectively the mid-points of sides AB, BC and CA of ΔABC. Find the ratio of the area of ΔDEF and ΔABC.
Sides of two similar triangles are in the ratio 4 : 9. Areas of these triangles are in the ratio
Triangles ABC and DEF are similar If AC = 19cm and DF = 8 cm, find the ratio of the area of two triangles.
Triangles ABC and DEF are similar If AB = 1.2 cm and DE = 1.4 cm, find the ratio of the areas of ΔABC and ΔDEF.
In ΔABC, D and E are the mid-points of AB and AC respectively. Find the ratio of the areas of ΔADE and ΔABC
The areas of two similar triangles are 121 cm2 and 64 cm2 respectively. If the median of the first triangle is 12.1 cm, find the corresponding median of the other.
The ratio of corresponding sides of similar triangles is 3 : 5; then find the ratio of their areas.
If ∆ABC ~ ∆PQR, A (∆ABC) = 80, A (∆PQR) = 125, then fill in the blanks. \[\frac{A\left( ∆ ABC \right)}{A\left( ∆ . . . . \right)} = \frac{80}{125} \therefore \frac{AB}{PQ} = \frac{......}{......}\]
In the given figure 1.66, seg PQ || seg DE, A(∆PQF) = 20 units, PF = 2 DP, then Find A(◻DPQE) by completing the following activity.

Prove that in a right triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides.
In ΔABC, PQ is a line segment intersecting AB at P and AC at Q such that seg PQ || seg BC. If PQ divides ΔABC into two equal parts having equal areas, find `"BP"/"AB"`.
In the given figure, ΔACB ~ ΔAPQ. If AB = 6 cm, BC = 8 cm, and PQ = 4 cm then AQ is equal to ______.

In a rhombus if d1 = 16 cm, d2 = 12 cm, its area will be ______.
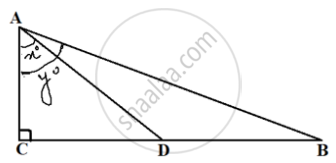
In the given figure, D is the mid-point of BC, then the value of `(coty^circ)/(cotx^circ)` is ______.
If the perimeter of two similar triangles is in the ratio 2 : 3, what is the ratio of their sides?
