Advertisements
Advertisements
Question
The band gap for silicon is 1.1 eV. (a) Find the ratio of the band gap to kT for silicon at room temperature 300 K. (b) At what temperature does this ratio become one tents of the value at 300 K? (Silicon will not retain its structure at these high temperatures.)
(Use Planck constant h = 4.14 × 10-15 eV-s, Boltzmann constant k = 8·62 × 10-5 eV/K.)
Solution
Given:-
Band gap of silicon, E = 1.1 eV
Temperature, T = 300 K
Boltzmann's constant, k = \[8 . 62 \times {10}^{- 5} e\]V/K
(a) We need to find out the ratio of the band gap to kT.
Ratio \[= \frac{1 . 1}{kT}\]
\[= \frac{1 . 1}{8 . 62 \times {10}^{- 5} \times 3 \times {10}^2}\]
\[ = 42 . 53 = 43\]
(b) The new ratio is `1/10` th of the earlier ratio.
i.e. New ratio = 4.253
We know,
Ratio = (Band gap)/(kT)
\[\Rightarrow 4 . 253 = \frac{1 . 1}{8 . 62 \times {10}^{- 5} \times T}\]
\[ \Rightarrow T = \frac{1 . 1}{8 . 62 \times {10}^{- 5} \times 4 . 253}\]
\[ \Rightarrow T = 3000 . 4 K \approx 3000 K\]
APPEARS IN
RELATED QUESTIONS
Draw the necessary energy band diagrams to distinguish between conductors, semiconductors and insulators.
How does the change in temperature affect the behaviour of these materials ? Explain briefly.
Draw separate energy band diagram for conductors, semiconductors and insulators and
label each of them.
Distinguish between a conductor, a semiconductor and an insulator on the basis of energy band diagrams.
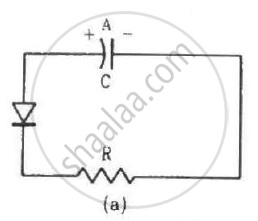
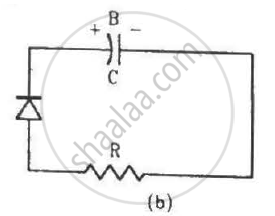
Two identical capacitors A and B are charged to the same potential V and are connected in two circuits at t = 0 as shown in figure. The charges on the capacitors at a time t = CRare, respectively,
The impurity atoms with which pure silicon may be doped to make it a p-type semiconductor are those of
(a) phosphorus
(b) boron
(c) antimony
(d) aluminium.
Suppose the energy liberated in the recombination of a hole-electron pair is converted into electromagnetic radiation. If the maximum wavelength emitted is 820 nm, what is the band gap?
(Use Planck constant h = 4.14 × 10-15 eV-s, Boltzmann constant k = 8·62 × 10-5 eV/K.)
The conductivity of an intrinsic semiconductor depends on temperature as σ = σ0e−ΔE/2kT, where σ0 is a constant. Find the temperature at which the conductivity of an intrinsic germanium semiconductor will be double of its value at T = 300 K. Assume that the gap for germanium is 0.650 eV and remains constant as the temperature is increased.
(Use Planck constant h = 4.14 × 10-15 eV-s, Boltzmann constant k = 8·62 × 10-5 eV/K.)
A semiconducting material has a band gap of 1 eV. Acceptor impurities are doped into it which create acceptor levels 1 meV above the valence band. Assume that the transition from one energy level to the other is almost forbidden if kT is less than 1/50 of the energy gap. Also if kT is more than twice the gap, the upper levels have maximum population. The temperature of the semiconductor is increased from 0 K. The concentration of the holes increases with temperature and after a certain temperature it becomes approximately constant. As the temperature is further increased, the hole concentration again starts increasing at a certain temperature. Find the order of the temperature range in which the hole concentration remains approximately constant.
(Use Planck constant h = 4.14 × 10-15 eV-s, Boltzmann constant k = 8·62 × 10-5 eV/K.)
Hydrogen atom in n = 3 state has a lifetime of 10-10 sec. The number of revolutions an electron makes in the n = 3 state before returning to the ground state is ______.
Useful data
`1/(4pi∈_0) = 8.99 xx 10^-34`N m2 C-2; e = 1.60 10-19 C; h = 6.63 10-34 Js; me = 9 × 10-3 kg
A window air conditioner is placed on a table inside a well-insulated apartment, plugged in and turned on. What happens to the average temperature of the apartment?
Two radioactive substances A and B have decay constants 3λ and λ respectively. At t = 0 they have the same number of nuclei. The ratio of the number of nuclei of A to those of B will be `1/"e"` after a time interval:
For germanium crystal, the forbidden gas energy gap
In a semiconductor, the forbidden energy gap between the valence, band and the conduction band is of the order of
The valance of an impurity added to germanium crystal in order to convert it into p-type semiconductor is
In a common base configuration Ie = 1 mA α = 0.95 the value of base current is
Three photo diodes D1, D2 and D3 are made of semiconductors having band gaps of 2.5 eV, 2 eV and 3 eV, respectively. Which 0 ones will be able to detect light of wavelength 6000 Å?
- Assertion (A): In insulators, the forbidden gap is very large.
- Reason (R): The valence electrons in an atom of an insulator are very tightly bound to the nucleus.
