Advertisements
Advertisements
Question
The class X students of a secondary school in Krishinagar have been allotted a rectangular plot of land for their gardening activity. Saplings of Gulmohar are planted on the boundary at a distance of 1 m from each other. There is a triangular grassy lawn in the plot as shown in the following figure. The students are to sow seeds of flowering plants on the remaining area of the plot.

(i) Taking A as origin, find the coordinates of the vertices of the triangle.
(ii) What will be the coordinates of the vertices of Δ PQR if C is the origin?
Also calculate the areas of the triangles in these cases. What do you observe?
Solution
(i) Taking A as origin, we will take AD as x-axis and AB as y-axis. It can be observed that the coordinates of point P, Q, and R are (4, 6), (3, 2), and (6, 5) respectively.
Area of triangle PQR = ` 1/2[x_1(y_2-y_3)+x_2(y_3-y_1)+x_3(y_1-y_2)]`
`= 1/2[4(2-5)+3(5-6)+6(6-2)]`
`=1/2[-12-3+24]`
=`9/2` square units
(ii) Taking C as origin, CB as x-axis, and CD as y-axis, the coordinates of vertices P, Q, and R are (12, 2), (13, 6), and (10, 3) respectively.
Area of triangle PQR = `1/2[x_1(y_2-y_3)+x_2(y_3-y_1)+x_3(y_1+y_2)]`
`=1/2[12(6-3)+13(3-2)+10(2-6)]`
`=1/2[36+13-40]`
`= 9/2 ` square units
It can be observed that the area of the triangle is same in both the cases.
APPEARS IN
RELATED QUESTIONS
Find the area of the following triangle:

Find the angle subtended at the origin by the line segment whose end points are (0, 100) and (10, 0).
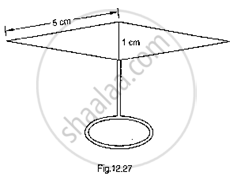
Find the area of the blades of thc magnetic compass shown in Fig.. 12.27. (Take √11 = 3.32).
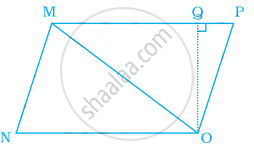
A(7, -3), B(5,3) and C(3,-1) are the vertices of a ΔABC and AD is its median. Prove that the median AD divides ΔABC into two triangles of equal areas.
If the points (2, -3), (k, -1), and (0, 4) are collinear, then find the value of 4k.
The area of the triangle whose vertices are A(1, 2), B(-2, 3) and C(-3, -4) is ______.
The area of ∆ABC is 8 cm2 in which AB = AC = 4 cm and ∠A = 90º.
Area of triangle MNO in the figure is ______.
In the given figure, ΔMNO is a right-angled triangle. Its legs are 6 cm and 8 cm long. Length of perpendicular NP on the side MO is ______.

Using determinants, find the area of ΔPQR with vertices P(3, 1), Q(9, 3) and R(5, 7). Also, find the equation of line PQ using determinants.
