Advertisements
Advertisements
Question
The constant A in the Richardson−Dushman equation for tungsten is 60 × 104 A m−2K−2. The work function of tungsten is 4.5 eV. A tungsten cathode with a surface area 2.0 × 10−5 m2 is heated by a 24 W electric heater. In steady state, the heat radiated by the heater and the cathode equals the energy input by the heater and the temperature becomes constant. Assuming that the cathode radiates like a blackbody, calculate the saturation current due to thermions. Take Stefan's Constant = 6 × 10−8 W m−2 K−1. Assume that the thermions take only a small fraction of the heat supplied.
Solution
Given:-
A = 60 × 104 A m−2 K−2
Work function of tungsten, ϕ = 4.5 eV
Stefan's Constant, σ = 6 × 10−8 W m−2 K−1
Surface area of tungsten cathode, S = 2.0 × 10−5 m2
Boltzmann's Constant, k = 1.38× 10−23 J/K
Heat supplied by the heater, H = 24 W
The cathode acts as a black body; thus, its emissivity is 1.
According Stefan's Law:-
The power (P) radiated by a blackbody with surface area (A) and temperature (T),
\[P = \sigma A T^4 \]
\[ \Rightarrow T^4 = \frac{P}{\sigma A}\]
\[ \Rightarrow T^4 = \frac{24}{(6 \times {10}^{- 8} ) \times (2 \times {10}^{- 5} )}\]
\[ \Rightarrow T^4 = 2 \times {10}^{13} K = 20 \times {10}^{12} K\]
\[ \Rightarrow T = 2 . 1147 \times {10}^3 = 2114 . 7 K\]
According to the Richard-Dushmann equation, emission current,
\[i = AS T^2 e^{- \phi/KT} \]
\[i = 6 \times {10}^5 \times 2 \times {10}^{- 5} \times (2114 . 7 )^2 \times e^\frac{- 4 . 5 \times 1 . 6 \times {10}^{- 19}}{1 . 38 \times {10}^{- 23} \times 2114 . 7} \]
\[i = 1 . 03456 \times {10}^{- 3} A \approx 1 . 0\text{ mA}\]
APPEARS IN
RELATED QUESTIONS
Find the maximum frequency of X-rays produced by 30 kV electrons.
Find the (a) maximum frequency, and (b) minimum wavelength of X-rays produced by 30 kV electrons.
How does one explain the emission of electrons from a photosensitive surface with the help of Einstein's photoelectric equation?
The work function of the following metals is given : Na 2.75 ev, K = 2.3 eV, Mo = 4.17 eV and Ni = 5.15 eV. Which of these metals will not cause photoelectric emission for radiation of wavelength 3300 Å from a laser source placed 1 m away from these metals? What happens if the laser source is brought nearer and placed 50 cm away?
How will the thermionic current vary if the filament current is increased?
An isolated metal sphere is heated to a high temperature. Will it become positively charged due to thermionic emission?
Let i0 be the thermionic current from a metal surface when the absolute temperature of the surface is T0. The temperature is slowly increased and the thermionic current is measured as a function of temperature. Which of the following plots may represent the variation in (i/i0) against (T/T0)?

The work function of aluminum is 4⋅2 eV. If two photons each of energy 2⋅5 eV are incident on its surface, will the emission of electrons take place? Justify your answer.
The wave associated with a moving particle of mass 3 × 10–6 g has the same wavelength as an electron moving with a velocity 6 × 106 ms–1. The velocity of the particle is
If a light of wavelength 330 nm is incident on a metal with work function 3.55 eV, the electrons are emitted. Then the wavelength of the wave associated with the emitted electron is (Take h = 6.6 × 10–34 Js)
Emission of electrons by the absorption of heat energy is called ____________ emission.
Why do metals have a large number of free electrons?
Define the work function of a metal. Give its unit.
What do you mean by electron emission? Explain briefly various methods of electron emission.
A 150 W lamp emits light of the mean wavelength of 5500 Å. If the efficiency is 12%, find out the number of photons emitted by the lamp in one second.
Photoelectric emission is observed from a metallic surface for frequencies ν1 and ν2 of the incident light (ν1 > ν2). If the maximum value of kinetic energy of the photoelectrons emitted in the two cases are in the ration 1 : n then the threshold frequency of the metallic surface is ______.
Consider Figure for photoemission.
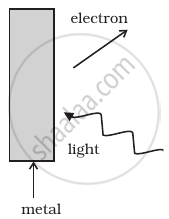
How would you reconcile with momentum conservation? Note light (photons) have momentum in a different direction than the emitted electrons.
Give an example each of a metal from which photoelectric emission takes place when irradiated by
- UV light
- visible light.
