Advertisements
Advertisements
Question
The coordinates of two points P and Q are (0,4) and (3,7) respectively. Find
(i) The gradient of PQ
(ii) the equation of PQ
(iii) the coordinates of the point where the line AB intersects the X-axis.
Solution

Slope of PQ = `(7 - 4)/(3 - 0)` = 1
(i) tan θ = 1
`therefore` Gradient = 1
(ii) Equation of PQ ⇒ `("y" - "y"_1)/("x" - "x"_1)` = slope
`("y" - 7)/("x" - 3)` = 1
⇒ x - 3 = y - 7
⇒ y = x + 4
(iii)
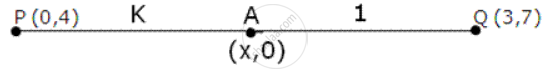
Let A (x,0) divides PQ is the ratio k : 1
Using section formula,
Coordinates of A (x,0) = `((3"k")/("k" + 1), (7"k" + 4)/("k" + 1))`
Equating we get
`(7"k" + 4)/("k" + 1)` = 0
7k + 4 = 0
APPEARS IN
RELATED QUESTIONS
Does the line 3x − 5y = 6 bisect the join of (5, −2) and (−1, 2)?
The co-ordinates of two points A and B are (–3, 4) and (2, –1). Find:
- the equation of AB;
- the co-ordinates of the point where the line AB intersects the y-axis.
The line through P (5, 3) intersects y-axis at Q.
write the equation of the line
A(1, 4), B(3, 2) and C(7, 5) are vertices of a triangle ABC. Find the equation of a line, through the centroid and parallel to AB.
Write down the equation of the line whose gradient is `3/2` and which passes through P, where P divides the line segment joining A(−2, 6) and B(3, −4) in the ratio 2 : 3.
Find if the following points lie on the given line or not:
(7, -2) on the line 5x + 7y = 11
Find the equation of a line whose slope and y-intercept are m = `(-6)/5`, c = 3
Find the equation of a line passing through (2,5) and making and angle of 30° with the positive direction of the x-axis.
Find the equation of a line passing through (-5,-1) and perpendicular to the 3x + y = 9
Find the value of ‘a’ for which the following points A (a, 3), B (2, 1) and C (5, a) are collinear. Hence find the equation of the line.
