Advertisements
Advertisements
Question
The experimental data for decomposition of N2O5
\[\ce{2N2O5 -> 4NO2 + O2}\] in gas phase at 318K are given below:
| t/s | 0 | 400 | 800 | 1200 | 1600 | 2000 | 2400 | 2800 | 3200 |
| 102 × [N2O5]/mol L−1 | 1.63 | 1.36 | 1.14 | 0.93 | 0.78 | 0.64 | 0.53 | 0.43 | 0.35 |
- Plot [N2O5] against t.
- Find the half-life period for the reaction.
- Draw a graph between log [N2O5] and t.
- What is the rate law?
- Calculate the rate constant.
- Calculate the half-life period from k and compare it with (ii).
Solution
(i)
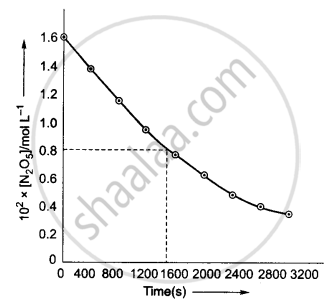
(ii) Initial concentration of N2O5 = 1.63 × 10−2 M
Half of this concentration = 0.815 × 10−2 M
The time corresponding to M concentration = 1440 s
Hence, `t_(1/2)` = 1440 s
(iii)
| t/s | [N2O5] × 102/mol L−1 | log [N2O5] |
| 0 | 1.63 | −1.79 |
| 400 | 1.36 | −1.87 |
| 800 | 1.14 | −1.94 |
| 1200 | 0.93 | −2.03 |
| 1600 | 0.78 | −2.11 |
| 2000 | 0.64 | −2.19 |
| 2400 | 0.53 | −2.28 |
| 2800 | 0.43 | −2.37 |
| 3200 | 0.35 | −2.46 |
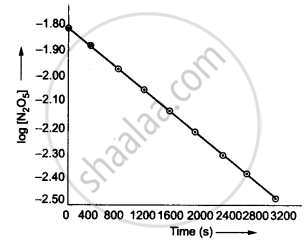
(iv) The given reaction is of the first order as the plot, log [N2O5] v/s t, is a straight line. Therefore, the rate law of the reaction is:
Rate = k[N2O5]
(v) For first order reaction,
log R = `- k/2.303t + logR_0`
Therefore the slope of the graph drawn between log R and t will be `(-k)/2.303`
∴ The slope of the line = `-k/2.303`
= `(y_2 - y_1)/(t_2 - t_1)`
= `(-2.46 - (-1.79))/(3200 - 0)`
= `-0.67/3200`
∴ k = 4.82 × 10−4 s−1
(vi) Half-life is given by,
`t_(1/2) = 0.639/k`
= `0.693/(4.82xx10^(-4))`
= 1.438 × 103 s
= 1438 s
This value, 1438 s, is very close to the value that was obtained from the graph.
APPEARS IN
RELATED QUESTIONS
The integrated rate equation for first order reaction is A → products
Derive the relation between half life and rate constant for a first order reaction
Sucrose decomposes in acid solution to give glucose and fructose according to the first order rate law. The half life of the reaction is 3 hours. Calculate fraction of sucrose which will remain after 8 hours.
For a first order reaction, show that time required for 99% completion is twice the time required for the completion of 90% of reaction.
A first order reaction takes 23.1 minutes for 50% completion. Calculate the time required for 75% completion of this reaction.
(log 2 = 0.301, log 3 = 0.4771, log 4 = 0.6021)
A first order reaction takes 30 minutes for 50% completion. Calculate the time required for 90% completion of this reaction.
(log 2 = 0.3010)
During nuclear explosion, one of the products is 90Sr with half-life of 28.1 years. If 1μg of 90Sr was absorbed in the bones of a newly born baby instead of calcium, how much of it will remain after 10 years and 60 years if it is not lost metabolically.
The rate constant for the first order decomposition of H2O2 is given by the following equation:
log k = 14.34 − 1.25 × 104 K/T. Calculate Ea for this reaction and at what temperature will its half-period be 256 minutes?
After 2 hours, a radioactive substance becomes `(1/16)^"th"` of original amount. Then the half life ( in min) is
The half life of the homogeneous gaseous reaction \[\ce{SO2Cl2 -> SO2 + Cl2}\] which obeys first order kinetics is 8.0 minutes. How long will it take for the concentration of SO2Cl2 to be reduced to 1% of the initial value?
For the first order reaction, half-life is equal to ____________.
Half life (t1/2) and completion time (T) of the zero order reaction are- (K = 0.001 mol/litre/sec and a = 1 M.)
A first-order reaction takes 69.3 min for 50% completion. What is the time needed for 80% of the reaction to get completed? (Given: log 5 = 0.6990, log 8 = 0.9030, log 2 = 0.3010)
The amount of C-14 isotope in a piece of wood is found to be 1/16th of its amount present in a fresh piece of wood. The age of wood, half-life period of C-14 is 5770 years, is ______ years.
For the given first order reaction A → B the half life of the reaction is 0.3010 min. The ratio of the initial concentration of reactant to the concentration of reactant at time 2.0 min will be equal to ______. (Nearest integer)
A reaction has a half-life of 1 min. The time required for 99.9% completion of the reaction is ______ min.
(Round off to the nearest integer).
[Use: In 2 = 0.69; In 10 = 2.3]
Assertion (A): The half-life of a reaction is the time in which the concentration of the reactant is reduced to one-half of its initial concentration.
Reason (R): In first-order kinetics, when the concentration of reactant is doubled, its half-life is doubled.
Obtain a relation, `k_2/k_1 = ((t_(1/2))_2)/((t_(1/2))_1)`, where k1 and k2 are rate constants while (t1/2)1 and (t1/2)2 are half-life periods of the first order reaction at temperatures T1 and T2 respectively. Write the relation for activation energy.
The half-lives of a first-order reaction are 1.19s at 313 K and 15.45s at 293 K. Calculate the energy of activation.
Calculate the half-life of a first order reaction from the rate constant given below:
2 min−1
