Advertisements
Advertisements
Question
The graph of y = 6 is a line ______.
Options
parallel to x-axis at a distance 6 units from the origin
parallel to y-axis at a distance 6 units from the origin
making an intercept 6 on the x-axis
making an intercept 6 on both the axes
Solution
The graph of y = 6 is a line parallel to x-axis at a distance 6 units from the origin.
Explanation:
Given equation of a line can be written as, 0 · x + l · y = 6
To draw the graph of above equation, we need atleast two solutions.
When x = 0, then y = 6
When x = 2, then y = 6
| x | 0 | 2 |
| y | 6 | 6 |
Hence, we find two points A(0, 6) and B(2, 6).
So, draw the graph by plotting these points and joining them, which is shown as follows:
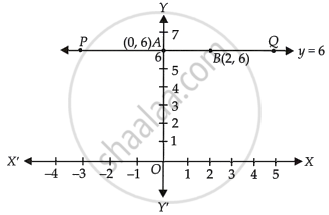
∴ The line PQ parallel to x-axis at a distance 6 units from origin is the required graph of y = 6.
APPEARS IN
RELATED QUESTIONS
If the point (3, 4) lies on the graph of the equation 3y = ax + 7, find the value of a.
From the choices given below, choose the equation whose graphs are given in the given figures.
For the first figure
(i) y = x
(ii) x + y = 0
(iii) y = 2x
(iv) 2 + 3y = 7x

For the second figure
(i) y = x +2
(ii) y = x − 2
(iii) y = − x + 2
(iv) x + 2y = 6

Draw the graph for the equation, given below :
x = 5
Draw the graph for the equation, given below :
5x + y + 5 = 0
Draw the graph (straight line) given by equation x - 3y = 18. If the straight line is drawn passes through the points (m, - 5) and (6, n); find the values of m and n.
Use the graphical method to find the value of k, if:
(5, k - 2) lies on the straight line x - 2y + 1 = 0
Use graph paper for this question. Take 2 cm = 2 units on x-axis and 2 cm = 1 unit on y-axis.
Solve graphically the following equation:
3x + 5y = 12; 3x - 5y + 18 = 0 (Plot only three points per line)
Draw the graphs of the following linear equations:
x = 3
Draw the graph for each of the following equation: Also, find the coordinates of the points where the graph of the equation meets the coordinate axes:
`(1)/(2) x + (1)/(3) y` = 1
Draw the graph of y = – 3x
