Advertisements
Advertisements
Question
The mid-point of the line segment joining (2a, 4) and (–2, 2b) is (1, 2a + 1). Find the values of a and b.
Solution
Mid-point of (2a, 4) and (−2, 2b) is (1, 2a + 1),
Therefore using mid-point formula, we have:
`x = (x_1 + x_2)/2 `
`1 = (2a - 2)/2`
1 = a − 1
a = 2
` y = (y_1 + y_2)/2`
`2a + 1 = (4 + 2b)/2`
2a + 1 = 2 + b
2 × 2 + 1 – 2 = b
b = 5 – 2 = 3
Therefore, a = 2, b = 3.
APPEARS IN
RELATED QUESTIONS
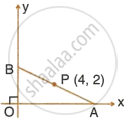
In the given figure, P(4, 2) is mid-point of line segment AB. Find the co-ordinates of A and B.
In the following example find the co-ordinate of point A which divides segment PQ in the ratio a : b.
P(–3, 7), Q(1, –4), a : b = 2 : 1
In the following example find the co-ordinate of point A which divides segment PQ in the ratio a : b.
P(–2, –5), Q(4, 3), a : b = 3 : 4
Find the midpoint of the line segment joining the following pair of point :
(4,7) and (10,15)
Find the midpoint of the line segment joining the following pair of point :
( a+3, 5b), (3a-1, 3b +4).
The mid-point of the line segment joining A (- 2 , 0) and B (x , y) is P (6 , 3). Find the coordinates of B.
A( 4, 2), B(-2, -6) and C(l, 1) are the vertices of triangle ABC. Find its centroid and the length of the median through C.
A(3, 1), B(y, 4) and C(1, x) are vertices of a triangle ABC. P, Q and R are mid - points of sides BC, CA and AB respectively. Show that the centroid of ΔPQR is the same as the centroid ΔABC.
O(0, 0) is the centre of a circle whose one chord is AB, where the points A and B are (8, 6) and (10, 0) respectively. OD is the perpendicular from the centre to the chord AB. Find the coordinates of the mid-point of OD.
Find coordinates of midpoint of segment joining (– 2, 6) and (8, 2)
