Advertisements
Advertisements
Question
The sides of a quadrilateral ABCD are 6 cm, 8 cm, 12 cm and 14 cm (taken in order) respectively, and the angle between the first two sides is a right angle. Find its area.
Solution
Given ABCD is a quadrilateral having sides AB = 6 cm, BC = 8 cm, CD = 12 cm and DA = 14 cm.
Now, join AC.
We have, ABC is a right-angled triangled at B.
Now, AC2 = AB2 + BC2 ...[By Pythagoras theorem]
= 62 + 82
= 36 + 64
= 100
⇒ AC = 10 cm ...[Taking positive square root]
∴ Area of quadrilateral ABCD = Area of ΔABC + Area of ΔACD ...(i)
Now, area of ΔABC = `1/2 xx AB xx BC` ...[∵ Area of triangle = `1/2` (base × height)]
= `1/2 xx 6 xx 8`
= 24 cm2
In ΔACD, AC = a = 10 cm, CD = b = 12 cm
And DA = c = 14 cm
Now, semi-perimeter of ΔACD,
`s = (a + b + c)/2`
= `(10 + 12 + 14)/2`
= `36/2`
= 18 cm
Area of ΔACD = `sqrt(s(s - a)(s - b)(s - c))` ...[By Heron’s formula]
= `sqrt(18(18 - 10)(18 - 12)(18 - 14))`
= `sqrt(18 xx 8 xx 6 xx 4)`
= `sqrt((3)^2 xx 2 xx 4 xx 2 xx 3 xx 2 xx 4)`
= `3 xx 4 xx 2 sqrt(3 xx 2)`
= `24sqrt(6) cm^2`
From equation (i),
Area of quadrilateral ABCD = Area of ΔABC + Area of ΔACD
= `24 + 24sqrt(6)`
= `24(1 + sqrt(6)) cm^2`
Hence, the area of quadrilateral is `24(1 + sqrt(6)) cm^2`.
APPEARS IN
RELATED QUESTIONS
An isosceles triangle has perimeter 30 cm and each of the equal sides is 12 cm. Find the area of the triangle.
Find the area of a triangle whose base and altitude are 5 cm and 4 cm respectively.
Determine whether the sets of points are collinear?
`(-1/2, 3), (-5, 6) and (-8, 8)`
If the points A(– 3, 9), B(a, b) and C(4, – 5) are collinear and if a + b = 1, then find a and b
The area of a triangle is 5 sq. units. Two of its vertices are (2, 1) and (3, −2). The third vertex is (x, y) where y = x + 3. Find the coordinates of the third vertex.
The sides of the triangular ground are 22 m, 120 m and 122 m. Find the area and cost of levelling the ground at the rate of ₹ 20 per m2
The cost of levelling the ground in the form of a triangle having the sides 51 m, 37 m and 20 m at the rate of Rs 3 per m2 is Rs 918.
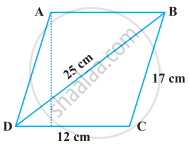
Find the area of a parallelogram given in figure. Also find the length of the altitude from vertex A on the side DC.
The perimeter of a triangle is 50 cm. One side of a triangle is 4 cm longer than the smaller side and the third side is 6 cm less than twice the smaller side. Find the area of the triangle.
A field is in the shape of a trapezium having parallel sides 90 m and 30 m. These sides meet the third side at right angles. The length of the fourth side is 100 m. If it costs Rs 4 to plough 1 m2 of the field, find the total cost of ploughing the field.
