Advertisements
Advertisements
Question
The total surface area of a cube is 216 cm2. Find its volume.
Solution
6(Edge)2 = Total surface area of a cube
6(Edge)2 = 216 cm2
=> (Edge)2 = `216/6`
=> (Edge)2 = 36
=> Edge = `sqrt(36)`
=> Edge = 6 cm
Volume of the given cube = (Edge)3 = (6)3 = 6 x 6 x 6 = 216 cm3
APPEARS IN
RELATED QUESTIONS
The length, breadth and height of a room are 5 m, 4 m and 3 m respectively. Find the cost of white washing the walls of the room and the ceiling at the rate of Rs 7.50 per m2.
A small indoor greenhouse (herbarium) is made entirely of glass panes (including base) held together with tape. It is 30 cm long, 25 cm wide and 25 cm high. What is the area of the glass?
Shanti Sweets Stall was placing an order for making cardboard boxes for packing their sweets. Two sizes of boxes were required. The bigger of dimensions 25 cm × 20 cm × 5 cm and the smaller of dimensions 15 cm × 12 cm × 5 cm. For all the overlaps, 5% of the total surface area is required extra. If the cost of the cardboard is Rs 4 for 1000 cm2, find the cost of cardboard required for supplying 250 boxes of each kind.
Daniel is painting the walls and ceiling of a cuboidal hall with length, breadth, and height of 15 m, 10 m, and 7 m, respectively. From each can of paint, 100 m2 of area is painted. How many cans of paint will she need to paint the room?
The length, breadth and height of a room are 5 m, 4 m and 3 m respectively. Find the cost
of white washing the walls of the room and the ceiling at the rate of Rs. 7.50 m2.
A cuboidal block of silver is 9 cm long, 4 cm broad and 3.5 cm in height. From it, beads of volume 1.5 cm3 each are to be made. Find the number of beads that can be made from the block.
A cuboidal block of solid iron has dimensions 50 cm, 45 cm and 34 cm. How many cuboids of size 5 cm by 3 cm by 2 cm can be obtained from this block? Assume cutting causes no wastage.
The volume of a cuboidal box is 48 cm3. If its height and length are 3 cm and 4 cm respectively, find its breadth.
Find the volume in cubic metre (cu. m) of the cuboid whose dimensions is length = 10 m, breadth = 25 dm, height = 50 cm.
A tank is 8 m long, 6 m broad and 2 m high. How much water can it contain?
A village, having a population of 4000, requires 150 litres water per head per day. It has a tank which is 20 m long, 15 m broad and 6 m high. For how many days will the water of this tank last?
An 8 m long cuboidal beam of wood when sliced produces four thousand 1 cm cubes and there is no wastage of wood in this process. If one edge of the beam is 0.5 m, find the third edge.
Find the surface area of a cuboid whoselength = 3.2 m, breadth = 30 dm, height = 250 cm.
The dimensions of a cuboid are in the ratio 5 : 3 : 1 and its total surface area is 414 m2. Find the dimensions.
A tank open at the top is made of iron sheet 4 m wide. If the dimensions of the tank are 12 m × 8 m × 6 m, find the cost of iron sheet at Rs 17.50 per metre.
If the areas of the adjacent faces of a rectangular block are in the ratio 2 : 3 : 4 and its volume is 9000 cm3, then the length of the shortest edge is
The dimensions of a Cinema Hall are 100 m, 60 m, and 15 m. How many persons can sit in the hall if each requires 150 m3 of air?
The external dimensions of a closed wooden box are 27 cm, 19 cm, and 11 cm. If the thickness of the wood in the box is 1.5 cm; find:
- The volume of the wood in the box;
- The cost of the box, if wood costs Rs. 1.20 per cm3;
- A number of 4 cm cubes that could be placed into the box.
Find the volume and the total surface area of a cuboid, whose :
l = 3.5 m, b = 2.6 m and h = 90 cm
Find the area of metal-sheet required to make an open tank of length = 10 m, breadth = 7.5 m and depth = 3.8 m.
The ratio between the curved surface area and the total surface area of a cylinder is 1: 2. Find the ratio between the height and the radius of the cylinder.
The dimensions of a cuboidal box are 6 m × 400 cm × 1.5 m. Find the cost of painting its entire outer surface at the rate of ₹ 22 per m2.
All six faces of a cuboid are ______ in shape and of ______ area.
The areas of any two faces of a cuboid are equal.
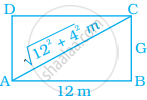
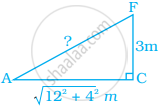
Find the length of the largest pole that can be placed in a room of dimensions 12 m × 4 m × 3 m.
Find the capacity of water tank, in litres, whose dimensions are 4.2 m, 3 m and 1.8 m?
