Advertisements
Advertisements
Question
The wheel of a motor cycle is of radius 35 cm. How many revolutions per minute must the wheel make so as to keep a speed of 66 km/hr ?
Solution
Given, radius of wheel, r = 35 cm
Circumference of the wheel
= 2πr
= `2 xx 22/7 xx 35 "cm"`
= 220 cm
But speed of the wheel
= 66 km/h–1
= `(66 xx 1000)/60` m/min
= 1100 × 100 cm/min–1
= 110000 cm/min–1
∴ Number of revolutions in 1 min
= `(110000)/220`
= 500 revolutions
Hence, the required number of revolutions per minute is 500.
APPEARS IN
RELATED QUESTIONS
A pendulum swings through an angle of 30º and describes an arc 8.8 cm in length. Find the length of the pendulum.
An arc of a circle is of length 5π cm and the sector it bounds has an area of 20 π cm2. Find the radius of the circle.
The radii of two circles are 8 cm and 6 cm respectively. Find the radius of the circle having area equal to the sum of the areas of the two circles.
In the given figure, the side of square is 28 cm and radius of each circle is half of the length of the side of the square where O and O' are centres of the circles. Find the area of shaded region.
The hypotenuse of a right-angled triangle is 65 cm and its base is 60 cm. Find the length of perpendicular and the area of the triangle.
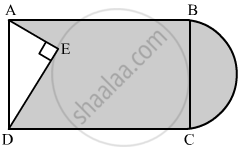
In the given figure, from a rectangular region ABCD with AB = 20 cm, a right triangle AED with AE = 9 cm and DE = 12 cm, is cut off. On the other end, taking BC as diameter, a semicircle is added on outside' the region. Find the area of the shaded region. [Use π = 3.14]
The area of a square is the same as the area of a square. Their perimeters are in the ratio
The radius of two circles are 20 cm and 13 cm. Find the difference between their circumferences. (Take π =`22/7`)
The diameter of a circular well is 4.2 m. What is its circumference?
Is it true that the distance travelled by a circular wheel of diameter d cm in one revolution is 2 π d cm? Why?
