Advertisements
Advertisements
Question
Triangle XYZ is right-angled at vertex Z. Calculate the length of YZ, if XY = 13 cm and XZ = 12 cm.
Solution
Given: ∆XYZ right angled at Z and XY = 13 cm, XZ = 12 cm.
To find: Length of YZ.
According to Pythagoras Theorem,
XY2 = XZ2 + YZ2
132 = 122 + YZ2
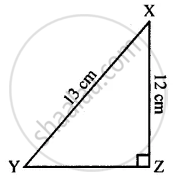
169= 144 +YZ2
169 − 144 = YZ2
25 = YZ2
∴ YZ = `sqrt25"cm"=sqrt(5xx5)` = 5 cm
APPEARS IN
RELATED QUESTIONS
P and Q are the mid-points of the sides CA and CB respectively of a ∆ABC, right angled at C. Prove that:
`(i) 4AQ^2 = 4AC^2 + BC^2`
`(ii) 4BP^2 = 4BC^2 + AC^2`
`(iii) (4AQ^2 + BP^2 ) = 5AB^2`
Two poles of heights 6 m and 11 m stand on a plane ground. If the distance between the feet of the poles is 12 m, find the distance between their tops.
D and E are points on the sides CA and CB respectively of a triangle ABC right angled at C. Prove that AE2 + BD2 = AB2 + DE2
A man goes 10 m due east and then 24 m due north. Find the distance from the straight point.
In the given figure, PQ = `"RS"/(3)` = 8cm, 3ST = 4QT = 48cm.
SHow that ∠RTP = 90°.
In triangle ABC, line I, is a perpendicular bisector of BC.
If BC = 12 cm, SM = 8 cm, find CS
The hypotenuse of a right angled triangle of sides 12 cm and 16 cm is __________
The perimeters of two similar triangles ABC and PQR are 60 cm and 36 cm respectively. If PQ = 9 cm, then AB equals ______.
Foot of a 10 m long ladder leaning against a vertical wall is 6 m away from the base of the wall. Find the height of the point on the wall where the top of the ladder reaches.
The longest side of a right angled triangle is called its ______.
