Advertisements
Advertisements
Question
Twelve wires each having a resistance of 3 Ω are connected to form a cubical network. A battery of 10 V and negligible internal resistance is connected across the diagonally opposite corners of this network. Determine its equivalent resistance and the current along each edge of the cube.
Advertisements
Solution
A battery of 10 V is connected across the diagonally opposite points a and f of the given network. Each side of the cube represents a 3-ohm resistor. Current entrees through the point a and leaves through the point f. Let I current is drawn from the battery and enters through point a, due to the symmetry of cube between point a and f, the current will divide itself into equal parts at each corner of the cube.
As you can see in the figure given below:
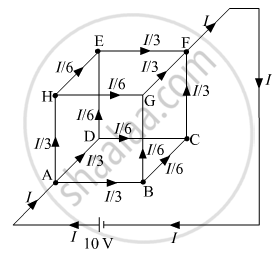
If we consider the current path ABGFA, then we can write, VA-B + VB-G + VG-F = V
⇒ `("I"/3 xx"R") + ("I"/6 xx "R") + ("I"/3 xx "R") = ("I" xx "R"_"net")`
⇒ `"R"_"net" = "R"/3 + "R"/6 + "R"/3 = (5"R")/6`
∵ R = 3Ω
⇒ `"R"_"net" = (5xx3)/6 = 2.5 Ω`
Current, `"i" = "V"/"R"_"net" = 10/2.5 = 4 "A"`
⇒ i = 4 A
As i = 4 A, hence current through each side of the cube can be marked. The below figure shows the current through each resistance of the network. i.e. each side of the cube.
APPEARS IN
RELATED QUESTIONS
State the two Kirchhoff’s rules used in electric networks. How are there rules justified?
Given n resistors each of resistance R, how will you combine them to get the (i) maximum (ii) minimum effective resistance? What is the ratio of the maximum to minimum resistance?
Determine the current drawn from a 12 V supply with internal resistance 0.5 Ω by the infinite network shown in the figure. Each resistor has 1 Ω resistance.
ε1 and ε2 are two batteries having emf of 34V and 10V respectively and internal resistance of 1Ω and 2Ω respectively. They are connected as shown in the figure below. Using Kirchhoff’s Laws of electrical networks, calculate the currents I1 and I2.
Consider the following two statements:-
(A) Kirchhoff's junction law follows from conservation of charge.
(B) Kirchhoff's loop law follows from conservative nature of electric field.
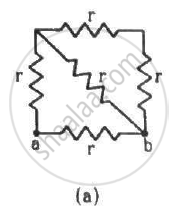
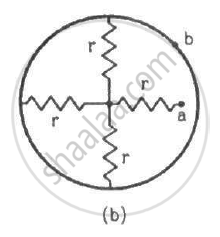
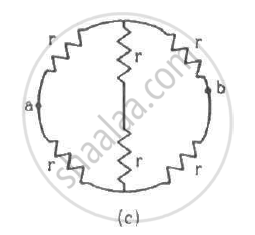
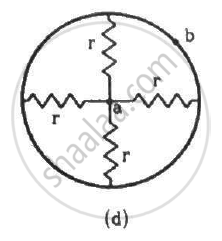
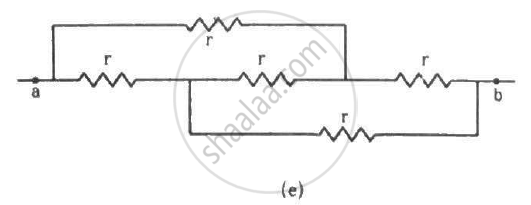
Twelve wires, each of equal resistance r, are joined to form a cube, as shown in the figure. Find the equivalent resistance between the diagonally-opposite points a and f.
Solve the following question.
Using Kirchhoff’s rules, calculate the current through the 40 Ω and 20 Ω resistors in the following circuit.
State Kirchhoff’s current rule.
In a potentiometer arrangement, a cell of emf 1.25 V gives a balance point at 35 cm length of the wire. If the cell is replaced by another cell and the balance point shifts to 63 cm, what is the emf of the second cell?
The instrument for the accurate measurement of the e.m.f of a cell is ______.
Figure shows current in a part of an electrical circuit. Then current I is ______.
Assertion: Kirchhoff’s junction rule follows from conservation of charge.
Reason: Kirchhoff’s loop rule follows from conservation of momentum.
While measuring the length of the rod by vernier callipers, the reading on the main scale is 6.4 cm and the eight divisions on vernier is in line with marking on the main scale division. If the least count of callipers is 0.01 and zero error - 0.04 cm, the length of the rod is ______.
Two cell of 1.25 V and 0.75 V are connected parallel. The effective voltage will be:-
Power P is to be delivered to a device via transmission cables having resistance RC. If V is the voltage across R and I the current through it, find the power wasted and how can it be reduced.
The value of current in the 6Ω resistance is ______.
Who gave Kirchhoff's laws?
What is a junction or node?
If currents \[I_1\] and \[I_2\] enter a junction and currents \[I_3\] and \[I_4\] leave it, which equation follows from Kirchhoff's First Law?
At junction P, currents \[0.2\,\mathrm{A}\], \[0.6\,\mathrm{A}\], and \[0.7\,\mathrm{A}\] point towards P, while \[0.4\,\mathrm{A}\], \[0.5\,\mathrm{A}\], and \[I\] point away. What is the unknown current?
