Advertisements
Advertisements
Question
Two identical current carrying coaxial loops, carry current I in an opposite sense. A simple amperian loop passes through both of them once. Calling the loop as C ______.
- `oint B.dl = +- 2μ_0I`
- the value of `oint B.dl` is independent of sense of C.
- there may be a point on C where B and dl are perpendicular.
- B vanishes everywhere on C.
Options
a and b
a and c
b and c
c and d
Advertisements
Solution
b and c
Explanation:
Ampere’s law gives another method to calculate the magnetic field due to a given current distribution.
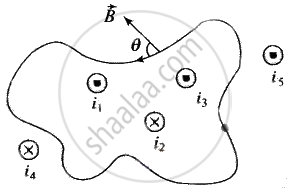
Line integral of the magnetic field `vecB` around any closed curve is equal to μ0 times the net current i threading through the area enclosed by the curve, i.e.
`oint vecB * vec(dl) = mu_0 sumi = mu_0 (i_1 + i_3 - i_2)`
Total current crossing the above area is `(i_1 + i_3 - i_2)`. Any current outside the area is not included in net current. (Outward ⊙ → + ve, Inward ⊗ → – ve)
Applying the Ampere's circuital law, we have
`ointB* dl = i_0 (I - I) = 0` (because current is in opposite sense)
Also, there may be a point on C where B and dl are perpendicular and hence, `oint_c B*dl = 0`
APPEARS IN
RELATED QUESTIONS
Write Maxwell's generalization of Ampere's circuital law. Show that in the process of charging a capacitor, the current produced within the plates of the capacitor is `I=varepsilon_0 (dphi_E)/dt,`where ΦE is the electric flux produced during charging of the capacitor plates.
State Ampere’s circuital law.
Electron drift speed is estimated to be of the order of mm s−1. Yet large current of the order of few amperes can be set up in the wire. Explain briefly.
A long, cylindrical wire of radius b carries a current i distributed uniformly over its cross section. Find the magnitude of the magnetic field at a point inside the wire at a distance a from the axis.
Two large metal sheets carry currents as shown in figure. The current through a strip of width dl is Kdl where K is a constant. Find the magnetic field at the points P, Q and R.
Calculate the magnetic field inside and outside of the long solenoid using Ampere’s circuital law
The wires which connect the battery of an automobile to its starting motor carry a current of 300 A (for a short time). What is the force per unit length between the wires if they are 70 cm long and 1.5 cm apart? Is the force attractive or repulsive?
Ampere’s circuital law is given by _______.
Two identical current carrying coaxial loops, carry current I in opposite sense. A simple amperian loop passes through both of them once. Calling the loop as C, then which statement is correct?
In a capillary tube, the water rises by 1.2 mm. The height of water that will rise in another capillary tube having half the radius of the first is:
A long solenoid having 200 turns per cm carries a current of 1.5 amp. At the centre of it is placed a coil of 100 turns of cross-sectional area 3.14 × 10−4 m2 having its axis parallel to the field produced by the solenoid. When the direction of current in the solenoid is reversed within 0.05 sec, the induced e.m.f. in the coil is:
Ampere's circuital law is used to find out ______
A thick current carrying cable of radius ‘R’ carries current ‘I’ uniformly distributed across its cross-section. The variation of magnetic field B(r) due to the cable with the distance ‘r’ from the axis of the cable is represented by:
Two concentric and coplanar circular loops P and Q have their radii in the ratio 2:3. Loop Q carries a current 9 A in the anticlockwise direction. For the magnetic field to be zero at the common centre, loop P must carry ______.
The given figure shows a long straight wire of a circular cross-section (radius a) carrying steady current I. The current I is uniformly distributed across this cross-section. Calculate the magnetic field in the region r < a and r > a.
Briefly explain various ways to increase the strength of the magnetic field produced by a given solenoid.
Using Ampere’s circuital law, obtain an expression for magnetic flux density ‘B’ at a point near an infinitely long and straight conductor, carrying a current I.
