Advertisements
Advertisements
Question
Use Einstein’s photoelectric equation to show how from this graph,
(i) Threshold frequency, and (ii) Planck’s constant can be determined.
Solution
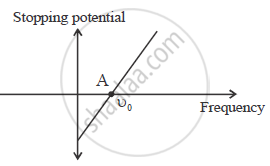
eV0 =hυ - hυ0
`V_0 = h/e(υ - v_0)`
Point A on the graph shows threshold frequency.
eV0 = hv - Φ0
`V_0 = h/e υ - (phi _0)/e`
Slope of the given graphs gives `h/e`
Slope `= h/e`
h = e × slope of the graph
APPEARS IN
RELATED QUESTIONS
In an experiment on the photoelectric effect, the slope of the cut-off voltage versus the frequency of incident light is found to be 4.12 × 10−15 Vs. Calculate the value of Planck’s constant.
In an accelerator experiment on high-energy collisions of electrons with positrons, a certain event is interpreted as annihilation of an electron-positron pair of total energy 10.2 BeV into two γ-rays of equal energy. What is the wavelength associated with each γ-ray? (1BeV = 109 eV)
The frequency and intensity of a light source are doubled. Consider the following statements.
(A) The saturation photocurrent remains almost the same.
(B) The maximum kinetic energy of the photoelectrons is doubled.
A non-monochromatic light is used in an experiment on photoelectric effect. The stopping potential
How does one explain the emission of electrons from a photosensitive surface with the help of Einstein’s photoelectric equation?
Choose the correct answer from given options
Photons of frequency v are incident on the surface of two metals A and B of threshold frequency 3/4 v and 2/3 v, respectively. The ratio of maximum kinetic energy of electrons emitted from A to that from B is
Each photon has the same speed but different ______.
The minimum energy required to remove an electron is called ______.
There are materials which absorb photons of shorter wavelength and emit photons of longer wavelength. Can there be stable substances which absorb photons of larger wavelength and emit light of shorter wavelength.
A photon of wavelength 663 nm is incident on a metal surface. The work function of the metal is 1.50 eV. The maximum kinetic energy of the emitted photoelectrons is ______.
