Advertisements
Advertisements
Question
Using Pythagoras theorem determine the length of AD in terms of b and c shown in Figure.
Solution
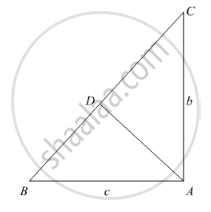
We have,
In ΔBAC, by Pythagoras theorem
BC2 = AB2 + AC2
⇒ BC2 = c2 + b2
⇒ BC = `sqrt(c^2+b^2)` ........(i)
In ΔABD and ΔCBA
∠B = ∠B [Common]
∠ADB = ∠BAC [Each 90°]
Then, ΔABD ~ ΔCBA [By AA similarity]
`therefore"AB"/"CB"="AD"/"CA"` [Corresponding parts of similar Δ are proportional]
`rArrc/sqrt(c^2+b^2)="AD"/b`
`rArr"AD"="bc"/sqrt(c^2+b^2)`
APPEARS IN
RELATED QUESTIONS
Construct a triangle ABC with sides BC = 7 cm, ∠B = 45° and ∠A = 105°. Then construct a triangle whose sides are `3/4` times the corresponding sides of ∆ABC.
If the sides of a triangle are 3 cm, 4 cm, and 6 cm long, determine whether the triangle is a right-angled triangle.
The sides of triangle is given below. Determine it is right triangle or not.
a = 9 cm, b = l6 cm and c = 18 cm
A triangle has sides 5 cm, 12 cm and 13 cm. Find the length to one decimal place, of the perpendicular from the opposite vertex to the side whose length is 13 cm.
ΔABC~ΔDEF such that ar(ΔABC) = 64 cm2 and ar(ΔDEF) = `169cm^2`. If BC = 4cm, find EF.
Find the length of each side of a rhombus are 40 cm and 42 cm. find the length of each side of the rhombus.
From given figure, In ∆ABC, AB ⊥ BC, AB = BC then m∠A = ?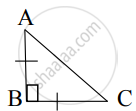
From given figure, In ∆ABC, AB ⊥ BC, AB = BC, AC = `2sqrt(2)` then l (AB) = ?
Find the height of an equilateral triangle having side 4 cm?
Find the altitude of an equilateral triangle of side 8 cm.
