Advertisements
Advertisements
Question
When the sun is directly overhead, the surface of the earth receives 1.4 × 103 W m−2 of sunlight. Assume that the light is monochromatic with average wavelength 500 nm and that no light is absorbed in between the sun and the earth's surface. The distance between the sun and the earth is 1.5 × 1011 m. (a) Calculate the number of photons falling per second on each square metre of earth's surface directly below the sun. (b) How many photons are there in each cubic metre near the earth's surface at any instant? (c) How many photons does the sun emit per second?
(Use h = 6.63 × 10-34J-s = 4.14 × 10-15 eV-s, c = 3 × 108 m/s and me = 9.1 × 10-31kg)
Solution
Here,
Intensity of light, `l = 1.4 × 10^3` W/m2,
Wavelength of light, `λ = 500 "nm" = 500 xx 10^-9 "m"`
Distance between the Sun and Earth, `l = 1.5 xx 10^11 "m"`
Intensity,
`I = "power"/"Area" = 1.4 xx 10^3 "W/m"^2`
Let n be the number of photons emitted per second.
∴ Power, P = Energy emitted/second
`P = (nhc)/λ`,
where λ = wavelength of light
h = Planck's constant
c = speed of light
Number of photons/`"m"^2` = `(nhc)/(λ xx A) = (nhc)/(λ xx 1) = l`
`therefore n = (I xx λ)/(hc)`
= `(1.4 xx 10^3 xx 500 xx 10^-9)/(6.63 xx 10^-34 xx 3 xx 10^8)`
= `3.5 xx 10^21`
(b) Consider number of two parts at a distance r and r + dr from the source.
Let dt' be the time interval in which the photon travels from one part to another.
Total number of photons emitted in this time interval,
`N = ndt = ((Pλ)/(hc xx A))(dr)/c`
These points will be between two spherical shells of radius 'r' and r + dr. It will be the distance of the 1st point from the sources.
In this case,
`l = 1.5 xx 10^11 "m"`
`"Wavelength" , λ = 500 "nm" = 500 xx 10^-9 "m"`
`P/(4pir^2) = 1.4 xx 10^3`
∴ No. of photons `/"m"^3 = P/(4pir^2) λ/(hc^2)`
= `1.4 xx 10^3 xx (500 xx 10^-9)/(6.63 xx 10^-34 xx 9 xx 10^16`
= `1.2 xx 10^13`
(c) Number of photons emitted = (Number of photons / s-m2) × Area
= `(3.5 xx 10^21) xx 4pil^2`
= `3.5 xx 10^21 xx 4 xx (3.14) xx (1.5 xx 10^11)^2`
= `9.9 xx 10^44`
APPEARS IN
RELATED QUESTIONS
A mercury lamp is a convenient source for studying frequency dependence of photoelectric emission, since it gives a number of spectral lines ranging from the UV to the red end of the visible spectrum. In our experiment with rubidium photo-cell, the following lines from a mercury source were used:
λ1 = 3650 Å, λ2 = 4047 Å, λ3 = 4358 Å, λ4 = 5461 Å, λ5 = 6907 Å,
The stopping voltages, respectively, were measured to be:
V01 = 1.28 V, V02 = 0.95 V, V03 = 0.74 V, V04 = 0.16 V, V05 = 0 V
Determine the value of Planck’s constant h, the threshold frequency and work function for the material.
[Note: You will notice that to get h from the data, you will need to know e (which you can take to be 1.6 × 10−19 C). Experiments of this kind on Na, Li, K, etc. were performed by Millikan, who, using his own value of e (from the oil-drop experiment) confirmed Einstein’s photoelectric equation and at the same time gave an independent estimate of the value of h.]
Draw graphs showing variation of photoelectric current with applied voltage for two incident radiations of equal frequency and different intensities. Mark the graph for the radiation of higher intensity.
What is the speed of a photon with respect to another photon if (a) the two photons are going in the same direction and (b) they are going in opposite directions?
In an experiment on photoelectric effect, a photon is incident on an electron from one direction and the photoelectron is emitted almost in the opposite direction. Does this violate the principle of conservation of momentum?
If an electron has a wavelength, does it also have a colour?
The work function of a metal is hv0. Light of frequency v falls on this metal. Photoelectric effect will take place only if
Light of wavelength λ falls on a metal with work-function hc/λ0. Photoelectric effect will take place only if
If the frequency of light in a photoelectric experiment is doubled, the stopping potential will ______.
In which of the following situations, the heavier of the two particles has smaller de Broglie wavelength? The two particles
(a) move with the same speed
(b) move with the same linear momentum
(c) move with the same kinetic energy
(d) have fallen through the same height
A beam of white light is incident normally on a plane surface absorbing 70% of the light and reflecting the rest. If the incident beam carries 10 W of power, find the force exerted by it on the surface.
(Use h = 6.63 × 10-34J-s = 4.14 × 10-15 eV-s, c = 3 × 108 m/s and me = 9.1 × 10-31kg)
A totally reflecting, small plane mirror placed horizontally faces a parallel beam of light, as shown in the figure. The mass of the mirror is 20 g. Assume that there is no absorption in the lens and that 30% of the light emitted by the source goes through the lens. Find the power of the source needed to support the weight of the mirror.
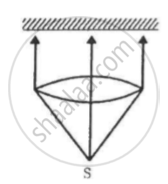
(Use h = 6.63 × 10-34J-s = 4.14 × 10-15 eV-s, c = 3 × 108 m/s and me = 9.1 × 10-31kg)
A sphere of radius 1.00 cm is placed in the path of a parallel beam of light of large aperture. The intensity of the light is 0.5 W cm−2. If the sphere completely absorbs the radiation falling on it, find the force exerted by the light beam on the sphere.
(Use h = 6.63 × 10-34J-s = 4.14 × 10-15 eV-s, c = 3 × 108 m/s and me = 9.1 × 10-31kg)
A sphere of radius 1.00 cm is placed in the path of a parallel beam of light of large aperture. The intensity of the light is 0.5 W cm−2. If the sphere completely absorbs the radiation falling on it, Show that the force on the sphere due to the light falling on it is the same even if the sphere is not perfectly absorbing.
The work function of a photoelectric material is 4.0 eV. (a) What is the threshold wavelength? (b) Find the wavelength of light for which the stopping potential is 2.5 V.
(Use h = 6.63 × 10-34J-s = 4.14 × 10-15 eV-s, c = 3 × 108 m/s and me = 9.1 × 10-31kg)
Answer the following question.
Plot a graph of photocurrent versus anode potential for radiation of frequency ν and intensities I1 and I2 (I1 < I2).
Define the terms "stopping potential' and 'threshold frequency' in relation to the photoelectric effect. How does one determine these physical quantities using Einstein's equation?
Two monochromatic beams A and B of equal intensity I, hit a screen. The number of photons hitting the screen by beam A is twice that by beam B. Then what inference can you make about their frequencies?
Why it is the frequency and not the intensity of the light source that determines whether the emission of photoelectrons will occur or not? Explain.
How would the stopping potential for a given photosensitive surface change if the intensity of incident radiation was decreased? Justify your answer.
How would the stopping potential for a given photosensitive surface change if the frequency of the incident radiation were increased? Justify your answer.
